RDP 9209: Financial Liberalisation and Consumption Behaviour 3. Cross-Country λ Comparisons and the Degree of Financial Liberalisation

One procedure for evaluating the PIH is to postulate a general model within which both the PIH and the Keynesian model (emphasising current income) are nested as special cases (as in Flavin (1985), Delong and Summers (1986), Jappelli and Pagano (1989), Campbell and Mankiw (1989) and Bayoumi and Koujianou (1989)). The significance of transitory income in explaining consumption is assumed to be evidence against one or all of the hypotheses listed in Section 2. This paper focuses on the last two of these, namely, no liquidity constraints and rational expectations, as the assumptions most likely to be responsible for the empirical rejection of the random walk consumption model.^[1]

Following Hayashi (1982) and Delong and Summers (1986), Jappelli and Pagano (1989) modify Hall's model of consumption to allow for a proportion of the population to be liquidity constrained. A first group of consumers represent that proportion of the total population who behave according to the RE-PIH and exhibit little if any sensitivity of consumption to transitory income. Jappelli and Pagano (J and P) assume quadratic utility, that is, they adopt equation (5) as their starting point, such that:

where:

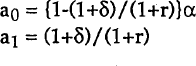
A second group of consumers represent that proportion, λ, of the total population who, perhaps because of liquidity constraints, consume all of their disposable income instead of obeying the RE-PIH:

Total per capita consumption is then:

Simple algebraic manipulation of equations (9), (10) and (11) yields an expression incorporating a non-linear constraint on the coefficient a₁:

The coefficient λ measures the degree of excess sensitivity of consumption to income. The model implies that this coefficient can also be interpreted as the share of income accruing to consumers who do not behave according to the RE-PIH.

Equation (12) is estimated using the technique of non-linear instrumental variables (NLIV). This circumvents the inconsistency problems associated with using Ordinary Least Squares to estimate a system of equations exhibiting correlated errors (the transitory consumption error, e_t in equation (12), is likely to be correlated with u_t in the disposable income equation (8)). The variables in X_t−1 of equation (8) are used as instruments. J and P use a constant term, a linear trend and the first lag of consumption, disposable income, government expenditure and exports. Estimated λ values may then be used to rank countries according to the degree of financial liberalisation, provided the estimates are based on the same definitions of regressors and instruments. Such comparisons also assume the degree of financial liberalisation has not been subject to change over time.

J and P estimate equation (12) on annual data for seven OECD countries. Their results are interesting in terms of the questions raised earlier. They find that their estimates of λ for each country have roughly the reverse ordering to various measures of consumer debt scaled by total consumption. This suggests that those countries with greater access to credit markets or, alternatively, those with a greater desire to use them, show a smaller proportion of the population that is liquidity constrained. Separate examination of a variety of demand side factors suggests that these do not explain the differences in λ values, leading the authors to conclude that capital market imperfections were the main factor.

These results are intuitively appealing. However, the approach appears to ignore the difficulties associated with estimating equation (12) in levels form; such estimation requires the assumption of trend stationarity of the regressors. This reservation with the model is echoed in Campbell and Mankiw (1991). The weight of evidence from other studies conducted over a reasonable time period is that at least some of J and P's regressors are non-stationary.^[2] West (1988) argues that, under certain circumstances, models containing one non-stationary regressor may still produce consistent and asymptotically normal estimators, provided that the regressor also exhibits non-zero drift. A selection of tests implemented on extended sample periods for two countries studied by J and P could not reject zero drift in a number of the non-stationary variables (see Appendix). It is also worth noting that J and P's use of annual data implies a very small number of observations (12 in the case of Japan and around 20 for most of their countries). This is not an adequate sample period to estimate their model. Nor do annual observations, which generate artificially smooth time averages of quarterly data, capture the notion of transitory income necessary to test the PIH.

Attempts to replicate and update J and P's non-linear instrumental variables estimation for a selection of three of their countries (the United States, Japan and Italy) were not satisfactory. For the United States and Japan, inconsistencies in the data were identified as the chief source of difference between the findings reported here and those of J and P (see Appendix for detailed estimation results and comparisons):

1. J and P define their consumption variable as total private consumption “...excluding expenditure on durables, defined as the sum of appliances, furniture and means of transportation” (p1102). This definition is applied to each of the countries in their sample with the exception of the United States. Their US data appear to exclude consumption expenditure on non-durable services. Correcting for this, the value of λ rises from 0.21 to 0.42, altering the ranking of the US from the second least liquidity-constrained country behind Sweden to the fourth (behind Sweden, Japan and the United Kingdom). This result throws the US out of line with their ranking in terms of debt to consumption. Furthermore, updating the sample period for the US from J and P's 1961–1984 to 1961–1990 leads to a rise in the λ parameter from 0.42 to 0.51. Since the period 1985 to 1990 encompassed increasing liberalisation and household borrowing, one might have expected the values of λ to fall.
2. In the case of Japan, J and P's results could only be replicated when national rather than personal disposable income, and total government outlays rather than the national accounts measure of government expenditure were used. Consequently, estimates for Japan using definitions consistent with those for the United States increased J and P's reported λ value of 0.34 to 0.51. This affects their ranking of Japan (making it the fourth rather than the third least liquidity-constrained country).

It did not prove possible to replicate J and P's results for Italy using the data sources referenced.

The sensitivity of the estimates of λ to precise definitions of the variables used for consumption, income and instruments, together with the above-mentioned econometric problems associated with using non-stationary variables and a small number of observations, led us to downplay the findings of J and P. This point can be illustrated intuitively with the specific country example of Sweden. Both J and P and Campbell and Mankiw (C and M) (1991) rank Sweden as the least liquidity-constrained country amongst those studied. This does not sit well with the fact that the radical liberalisation of Sweden's financial markets was delayed until the second half of the 1980s (certainly outside of J and P's sample period), suggesting that other country-specific factors might have influenced the estimate of λ (for example, unique social security arrangements).

Similarly, important changes in the degree of financial liberalisation occurred in a number of the countries studied by J and P and C and M in the second half of the 1970s and throughout the 1980s. This is ignored by J and P and is touched on relatively briefly by C and M. The latter test whether λ has been a linear function of time, or was subject to a structural break in 1980, and find little (though not zero) support for either proposition. This dismissal of time variation in the responsiveness of consumption to income could, however, be due to the very specific nature of the tests carried out by C and M. Thus λ is unlikely to have been a linear function of time prior to the major deregulatory moves. With regard to their dummy variable test, C and M do not permit the drift parameter to change, and they impose the value of the co-efficient used to weight current and lagged changes in income. By forcing the intercept coefficient to be the same in both sub-periods, their estimates of λ may be biased.

For these reasons, and given the difficulties with cross-country comparisons, further exploration of the extent to which λ may have varied over time in response to financial liberalisation measures seems warranted.

Footnotes

Augmenting the nested specification by including a variable which causes consumers to experience liquidity constraints may permit the data to reject one or other of these competing explanations. Flavin suggests that the unemployment rate is such a variable, since it can be interpreted as a proxy for the proportion of the population subject to liquidity constraints. Although a much greater fraction of the population than those who are unemployed probably experience liquidity constraints, nevertheless an increase in unemployment will increase the fraction of the population who are constrained to consume from current disposable resources. The full gamut of tests presented in this paper for a simpler model (focusing on current income) was also applied to the Flavin model. Since the overall conclusions based on the two models are essentially no different, it was decided not to work with the Flavin model, which proved somewhat unwieldly for examining some of the issues raised. [1]

Existing tests for non-stationarity cannot be applied to J and P's data as the tests' powers are particularly low over small samples containing less than 100 observations. Nevertheless, augmented Dickey-Fuller and Phillips-Perron tests are applied to extended sample periods for two of the seven countries investigated by J and P. The results are presented in the Appendix. The small sample periods over which the non-linear estimation is performed (e.g. 12 observations for Japan, and an average of about 20 for other countries) poses problems for these tests of the individual variables, and certainly for the adequate estimation of J and P's model. [2]