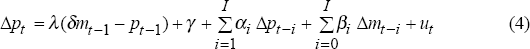RDP 9707: Internationalisation and Pricing Behaviour: Some Evidence for Australia 4. Openness and the Dynamic Response of Domestic Prices to Changes in Import Prices

James O'Regan, Jenny Wilkinson

October 1997

There are two main channels through which changes in imported goods prices affect the domestic price level. Both of these are likely to be affected by openness.

Changes in import prices affect the domestic price level directly through the prices of imported final goods which are consumed in the economy.[26] The importance of this channel clearly depends on the share of imported goods in the bundle of goods consumed domestically. As discussed in Gruen and Sheutrim (1994), if increasing the openness of the economy leads to a higher proportion of imported goods in the consumption bundle, it should also lead to a commensurate increase in the impact that a given change in the price of imported goods (or, similarly, a change in the exchange rate) has on the aggregate inflation rate.

Changes in import prices also affect the domestic price level indirectly through the prices set for domestically produced goods. This occurs in two ways. First, to the extent that domestic industries use imported intermediate inputs in production, increases in import prices may be passed on to domestic prices. Second, since relative prices matter, domestic price setters in import-competing industries may be sensitive to import prices. The degree of sensitivity of domestic price setters to import prices depends on the substitutability of goods and the openness of markets. While most tradeable goods are not perfect substitutes for each other, opening up the economy should nonetheless deepen the market for categories of goods which have similar attributes, and should increase the sensitivity of domestic price setters to changes in the prices of their imported substitutes.

In this section we focus on the indirect channel through which import prices affect the domestic price level and examine how it depends on the degree of openness of the economy. We start by examining the different aggregate responses of domestically produced tradeable and non-tradeable goods prices to changes in import prices. We then turn to more disaggregated industry-level data on the prices of domestically produced import-competing goods to identify the role of openness in this relationship.

4.1 Tradeable and Non-tradeable Goods Prices and Import Prices

An initial assessment of the impact that changes in openness may have on price-setting behaviour can be made by comparing the responses to changes in import prices of domestic industries which primarily produce traded goods with those which primarily produce non-traded goods. Prices in both types of industries would be expected to respond to import prices to the extent that intermediate imported goods are used in production. Given that tradeable goods are presumably closer substitutes with the imported goods, however, their sensitivity to changes in imported goods prices would be expected to be greater.

To examine the response of domestic producer prices to import prices, we make use of producer price indices recently published by the ABS for the non-tradeable, tradeable and importable sectors of the domestic economy.[27] Year-ended changes in these indices are shown along with changes in import prices in Figure 3. This figure illustrates that tradeable and importable prices move more closely with imported prices than do non-tradeable prices, though common long-term trends are evident for all three series.

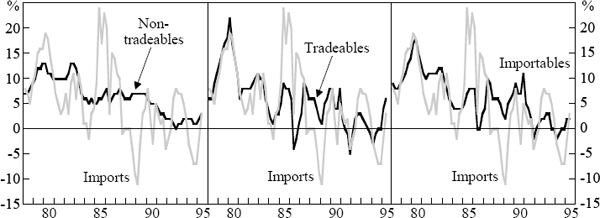
Figure 3: Prices of Domestically Produced Goods and Import Prices
Year-ended percentage change
Figure 3: Prices of Domestically Produced Goods and Import Prices

To examine these relationships more rigorously, we estimate an error-correction model for each sector, and on the basis of this, derive both the elasticity of domestic prices with respect to import prices in each sector and the speed of response of domestic prices to a shock to import prices. That is, for each sector we estimate a model of the following form:

where pt is the price of the domestically produced good in a sector, mt is the price of imported goods and all prices are expressed in logs.[28] The following results are based on the most parsimonious equations estimated using a general-to-specific modelling strategy using up to eight lags on the first difference terms.

In this exercise, we have not taken into account the way any variables other than import prices affect domestic prices. This is because we want to capture the total impact that changes in import prices have on domestic prices. Changes in costs may reflect changes in import prices to the extent that costs include the prices of imported intermediate inputs and to the extent that there are some components of costs, in particular wages or productivity, which may vary in response to changes in the international environment. We wish to attribute these indirect effects to the total impact of changes in import prices.

This approach will overstate the influence of import prices, however, to the extent that imported goods prices are endogenous to changes in other costs in the domestic economy. For example, a positive wages shock would be expected to lead to an exchange rate depreciation and hence, an increase in imported goods prices. The long-run response of domestic prices (both tradeable and non-tradeable) to import prices, will thus in part reflect the fact that, in history, the exchange rate has responded to such exogenous inflationary shocks.

A summary of the results from these regressions is presented in Table 3. For each sector, this table shows the estimated long-run elasticity (δ) and speed-of-adjustment coefficient (λ) over three sample periods.

Table 3: Sectoral Responses to Import Prices(a)
1977:Q1–1995:Q2 1977:Q1–1989:Q2 1984:Q2–1995:Q2
Sector
 		 Long-run elasticity Speed of adjustment Long-run elasticity Speed of adjustment Long-run elasticity Speed of adjustment
Non-tradeables 0.85
(0.08)		 0.05
(0.01)		 0.84
(0.07)		 0.05
(0.01)		 0.93
(0.11)		 0.04
(0.01)
Tradeables 0.72
(0.05)		 0.12
(0.02)		 0.67
(0.04)		 0.14
(0.03)		 0.76
(0.08)		 0.11
(0.03)
Importables 0.87
(0.07)		 0.08
(0.02)		 0.80
(0.05)		 0.10
(0.03)		 0.96
(0.14)		 0.07
(0.02)
Joint significance of tradeables and
non-tradeables(b)		 2.74
[0.10]		   5.96
[0.01]		   2.04
[0.15]		  
Notes: (a) These equations have been estimated within a SUR system to allow for cross-equation correlations in errors. Numbers in parentheses ( ) are standard errors. Numbers in brackets [ ] are p-values. For the long-run elasticity estimates, standard errors have been derived using a Bewley transformation.
(b) Chi-squared test of the cross-equation restriction that the non-tradeables and tradeables coefficient estimates are equal. The p-value gives the level of significance at which one can reject the null hypothesis.

Over the full sample period, these results suggest that the long-run responses of the non-tradeable and importable sectors to a change in import prices are high; they are both insignificantly different from 1 at a one per cent level of significance. The long-run response of all tradeable prices to import prices is lower, presumably reflecting the different composition of our importable and exportable sectors and the understandably lower response of exportable prices to import prices.

These sectors differ more markedly from each other, however, in the speed of adjustment to disequilibrium between imported and domestic prices. Prices in the tradeables sector respond over twice as rapidly as non-tradeable prices, and also somewhat faster than importables prices. One year after a shock to import prices, non-tradeable prices have made under 20 per cent of the total adjustment to the import price shock, while tradeable prices have adjusted by around 40 per cent. It is somewhat puzzling that importables respond less rapidly to a shock to import prices than exportables and importables taken together. This may reflect the fact that exchange rate shocks (which are the major source of shocks to import prices) are more quickly passed through to many of our export prices, because the bulk of our exports are homogeneous commodities whose prices are essentially set on world markets. The importable sector, in contrast, comprises a larger proportion of differentiated goods, which do not tend to be as sensitive, over the short term, to a divergence between the prices of imported and domestically produced items.

Since the degree of import penetration has increased over the past two decades, a further assessment of the impact of openness on price setting behaviour can be made by examining these relationships over two sub-samples. The results of this exercise, reported in the second two panels in Table 3, are somewhat mixed. The speed of response of domestic tradeable prices to import prices does not appear to have increased over the two sample periods, and although the long-run response of domestic prices to import prices has increased in each of the sectors, standard errors on the estimates for the second sample period are sufficiently large to preclude any strong conclusions being drawn.

In summary, given that the tradeable sector is by definition more open than the non-tradeable sector, these results provide evidence that openness increases the sensitivity of domestic prices to variations in world prices and the exchange rate.

4.2 Openness and Industry Level Responses to Import Prices

We now turn to disaggregated industry-level data for import-competing manufacturing industries to better discern the differential impact that openness may have within tradeable industries.

There has, to our knowledge, been no study which has examined at a disaggregated level the relationship between import prices and the prices set by domestic producers of import-competing goods in Australia since Gregory (1978). Gregory used disaggregated industry-level data over the period from 1970 to 1974 to assess how relative price changes across industries could be related to relative import price changes, relative cost changes and changes in the pattern of demand. He found that relative import price changes could not explain relative price changes; domestic producers appeared to adjust their market share rather than their price when faced with a change in the price of imports.[29]

In this section of the paper we examine the sensitivity of domestic producers of import-competing goods to the prices of their imported substitutes, using disaggregated data for the manufacturing industry.[30] For each of 30 industry groups, we match quarterly data on the prices of domestically produced goods and imported goods over the period from June 1983 to June 1995.

A preliminary look at the data, using simple correlation analysis, is provided in Table 4. This shows the contemporaneous correlation between quarterly percentage changes in import prices and domestic prices for each industry. These correlation coefficients have been averaged over all of the industries in a given group. The degree of international exposure of each industry is measured by the import share, and for the purposes of this table, the industries have been divided into three roughly even sized groups.

Table 4: Correlation between Quarterly Changes in Import Prices and Domestic Prices(a)
All industries Degree of international exposure(b)
Low Medium High
1983:Q3–1995:Q2 0.18 0.10 0.19 0.25
1983:Q3–1989:Q2 0.13 0.02 0.15 0.23
1989:Q3–1995:Q2 0.19 0.13 0.17 0.26
Notes: (a) Simple averages of the correlation between quarterly percentage changes in imported and domestically produced goods prices in each industry.
(b) Based on the average level of the import share over the period 1983–1992. ‘Low’ industries have an import share less than 20 per cent, ‘medium’ industries have an import share between 20 and 39 per cent, and ‘high’ industries have an import share above 39 per cent.

This table suggests that the correlation between import price changes and domestic price changes is positively related to the degree of international exposure of the industry; those industries which have higher import shares also have a consistently higher correlation between changes in import prices and changes in domestic prices. Across all industries and each sub-group of industries, the average correlation has also been higher over the second half of the sample than the first half. Given that the degree of international exposure has increased in all but three of the industries we examined, this is also suggestive of a positive relationship between openness and the sensitivity of domestic producers to import prices.

The average correlation coefficients quoted above, however, are relatively small. In part, this is because this analysis only captures the contemporaneous correlation between the two series and is thus an incomplete description of the underlying relationship.

To look more comprehensively at these relationships, we estimate error-correction models for each industry, using the same structure as Equation (4).[31] Estimates of the long-run elasticities of domestic prices to import prices within each industry are presented in Table 5.[32] This shows that for 27 of the 30 industries, the long-run elasticity estimates are significantly greater than zero, and for 21 of these industries, the elasticities are significantly less than one. That these elasticities are significant, but less than one, is consistent with the results in Table 3. It is also consistent with the conclusion from Table 1 that domestic prices have been increasing less rapidly than foreign prices in many manufacturing industries. For these 27 industries, the estimated elasticities range between 0.33 and 1.15, and average 0.67.

Table 5: Industry Estimates of Long-run Import Price Elasticity and Speed of Adjustment(a)
Industry Long-run elasticity Speed of adjustment
Point
estimate		 t-statistic Point
estimate		 t-statistic
Fruit and vegetables 0.36 1.89 0.016 2.77
Margarines, oils and fats −0.04 −0.47 0.025 0.82
Alcohol 0.61 11.99 0.044 3.71
Textiles 0.86 15.29 0.083 6.38
Clothing 0.81 4.08 0.019 3.49
Footwear 0.62 7.66 0.037 3.07
Sawmill products 0.63 30.01 0.105 2.77
Woodboards, veneers and joinery 0.77 12.85 0.052 4.14
Furniture and mattresses 0.62 5.41 0.030 4.39
Pulp, paper and paperboard 0.89 10.38 0.032 1.16
Chemical fertilisers −0.35 −3.39 0.035 2.28
Basic chemicals 0.77 17.90 0.041 0.98
Pharmaceutical and veterinary products; pesticides 0.45 3.56 0.027 3.38
Cosmetics and toilet preparations 0.44 9.80 0.050 1.71
Petroleum and coal products 0.63 15.98 0.090 1.29
Glass and glass products 0.43 11.82 0.069 2.77
Clay products 0.35 6.02 0.018 2.86
Structural products, sheet metal 0.55 9.47 0.042 3.92
Motor vehicles and parts 0.64 18.88 0.059 4.82
Photographic, professional and scientific equipment 0.33 11.62 0.126 2.56
Electronic equipment 0.55 6.65 0.040 1.34
Refrigerators, household 0.65 6.65 0.056 4.33
Electrical equipment n.e.c. 1.15 6.42 0.031 3.09
Agricultural machinery(b) 1.15 4.32 0.072 3.59
Construction machinery 0.98 12.28 0.078 2.87
Machinery and equipment n.e.c. 0.44 11.22 0.055 3.02
Leather products 0.65 12.43 0.105 2.34
Rubber products 0.96 12.76 0.061 4.55
Signs, advert displays; writing equipment 0.99 19.38 0.098 5.29
Manufacturing n.e.c. 0.65 9.56 0.067 3.10
Notes: (a) Estimated by SUR. The industries (excluding agricultural machinery) were divided into three groups, and each group was estimated as a system. The distribution of the t-statistics on the speed-of-adjustment coefficients are between a standard normal and Dickey-Fuller distributions. The distribution of t-statistics on the long-run elasticity estimates (derived using a Bewley transformation) are standard.
(b) Estimated by OLS.

Table 5 also presents estimates of the speed-of-adjustment coefficient for each industry. These are significant in all but 6 industries, suggesting that domestic and imported goods prices are linked together over the long-run. The coefficient estimates are, however, relatively low, ranging between 0.02 and 0.13, suggesting that there can be substantial deviations from this long-run relationship through time.[33] Interestingly, the speed-of-adjustment coefficients presented in Table 5 are substantially lower than those presented in Table 3 for the tradeables industries, though they are close to those presented for the importables industries. This may be because, at a disaggregated level, industry-specific factors are more important, making the underlying relationship harder to identify.[34]

The wide range of estimated elasticities across industries may reflect a number of factors. It may reflect the fact that some industries are more open than others, and hence, are more sensitive to changes in import prices. Alternatively, it may reflect the fact that the data are not sufficiently disaggregated, and the composition of the domestically produced goods vary significantly from the goods which are imported. In this case, one would not expect the domestic price series to respond fully to changes in import prices even if the law of one price held. Finally, it may reflect a tendency towards price stickiness on the part of some groups of domestic producers, and suggest that producers are still prepared to concede market share to imports when the price of imports fall.

To examine the role of openness in driving the differential results across industries, we examine the relationship between the estimated elasticities and the industry import shares. Figure 4 suggests that there is little relationship between the degree of openness of an industry, as measured by the import share, and the elasticities; econometric estimation confirms this.[35]

Figure 4: Import Price Elasticities and Openness
Figure 4: Import Price Elasticities and Openness

The relationship between the speed-of-adjustment coefficients and the degree of openness of the industries is presented in Figure 5. This figure shows a much clearer positive relationship. A regression of the industry speed-of-adjustment estimates against the degree of openness of the industry finds that this relationship is significant.[36]

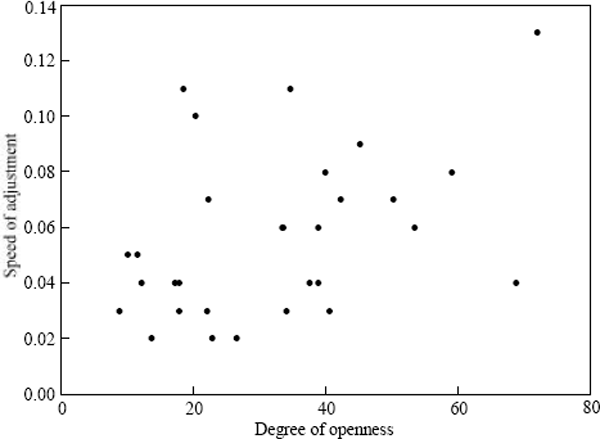
Figure 5: Speed of Adjustment and Openness
Figure 5: Speed of Adjustment and Openness

Our interpretation of the combined elasticity and speed-of-adjustment results is as follows. For a given degree of openness of an industry, the long-run response of domestic prices to import prices reflects the composition and substitutability of domestically produced and imported goods. The more open the industry, however, the faster domestic price setters will respond to any change in the price of the imported goods with which they compete. More open industries thus do appear to be more sensitive to the prices of foreign-produced substitute goods, in the sense that they respond more rapidly to a change in the price of these goods than do less open industries.[37]

Footnotes

Dwyer and Lam (1994) examine the relationship between the over-the-docks (wholesale) price of imports and the retail price. They find that changes in wholesale import prices are fully passed through to retail import prices, though taking into account domestic add-on costs, the retail import price moves by around two thirds of the change in the wholesale price. [26]

These indices have been compiled by Johnson (1996), broadly following the methodology developed in Dwyer (1990,1992). Industries are classified as tradeable if either their import share or their export share is greater than 10 per cent and are classified as importable if their import share is greater than 10 per cent. For this study we use the chained tornqvist indices calculated by Johnson. [27]

The price of imported goods is measured by the implicit price deflator for imported goods. [28]

For his central results, Gregory used a panel regression in which he restricted the response of domestic prices to import prices to be the same across industries. When he allowed some variation across industries based on the degree of import competition, he did find a significant positive response among the more open industries, although other explanators remained much more important for explaining movements in domestic prices. [29]

Industries were included if they had been import competing at some time during the sample period. [30]

All import price series are tariff adjusted using the average duty rates series compiled from ABS data. For further details on data sources and series construction, see Appendix C. There is little difference between the results obtained using tariff-adjusted and unadjusted data. [31]

The results presented and discussed here are estimated using the seemingly unrelated regression (SUR) technique. Applying this technique improves the precision of the coefficient estimates by taking into account the correlation of errors across equations. Estimates based on OLS regressions are similar, and are presented in Appendix B. [32]

On average, these coefficients imply that only half of the response of domestic prices to a shock to import prices takes place in two and a half years. [33]

Measurement error in the disaggregated data could also bias the speed-of-adjustment coefficient estimates toward zero. To the extent that these errors are uncorrelated across industries, they would be smaller in the aggregated data. [34]

The cross-section regression results are as follows: 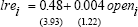Inline Equation, where lrei is the long-run elasticity estimate for industry i, openi is the import share for industry i, and t-statistics are presented in parentheses. If this cross-section regression is restricted to those industries in which a significant long-run relationship between domestic and imported prices is found, the results are essentially unchanged. We attempted to control for the concentration of the industry by including either the concentration of the industry, or the interaction between concentration and openness, however these explanators were insignificant. [35]

The cross-section regression results are as follows: 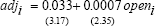Inline Equation, where adji is the coefficient on the error-correction term (or speed-of-adjustment coefficient) for industry i, openi is the import share for industry i, and t-statistics are presented in parentheses. This relationship is stronger if only industries in which a significant long-run relationship between domestic and import prices exists are included. Controlling for the different concentration ratios across industries makes little difference to the results. [36]

We examined whether the responsiveness of domestic prices to import price changed over the sample period by splitting the sample into two, and comparing both the long-run elasticities and speed of adjustment across sample periods. The shorter sample periods substantially reduced the statistical significance of the estimates, however, precluding any conclusions being drawn. [37]