RDP 2004-10: News and Interest Rate Expectations: A Study of Six Central Banks 4. Measuring the Impact of News on Interest Rates: A Cross-country Study
November 2004
- Download the Paper 886KB
Empirical modelling of financial time-series data usually needs to take account of changing asset return variance, whereby periods of low and high volatility tend to be clustered. This phenomenon can be captured by employing models of conditional heteroskedasticity such as the ARCH (autoregressive conditional heteroskedasticity) and GARCH (generalised ARCH) models suggested by Engle (1982) and Bollerslev (1986). As mentioned above, such an approach allows us to deal with the different nature of the central bank communication variable compared with macroeconomic and monetary policy surprises. It does so by simultaneously estimating the mean equation for interest rate futures and the variance of the residuals from the mean equation.
The next section briefly describes the specific model estimated, using the data described in Section 3.1. In Section 4.2 and Section 4.3 we present the empirical results for the effect of different types of news: domestic macroeconomic data releases, foreign market movements, monetary policy surprises, and different channels of central bank communication. Comparing the results across different economies also allows us to assess the effectiveness of these channels across different monetary policy frameworks.
4.1 The Econometric Model
The econometric model underlying our analysis of interest rate futures is an EGARCH (exponential generalised autoregressive conditional heteroskedasticity) model suggested by Nelson (1991). The exponential form allows for asymmetry in the response of interest rate futures following positive or negative shocks. It has the added advantage of guaranteeing that the estimated daily conditional variance is always positive.[9]
4.1.1 The mean equation
The mean equation for changes in 90-day bank bill futures rates, Δft, is specified for each economy as in Equation (1), but we exclude central bank communication events:
4.1.2 The variance equation
To explicitly model ARCH effects, we assume that the residuals from the mean Equation (3) can be modelled as a function of the standard deviation of the residuals ht, and an independently and identically distributed term νt:
νt are also known as the standardised residuals:
The variance of the residuals,  , is
modelled as a function of its own past values, past errors from the mean equation and other
factors which may be influencing the conditional variance.[10] In our EGARCH(x,y)
framework, we assume that the logged variance
, is
modelled as a function of its own past values, past errors from the mean equation and other
factors which may be influencing the conditional variance.[10] In our EGARCH(x,y)
framework, we assume that the logged variance  of the residuals can
be modelled as:
of the residuals can
be modelled as:
where comw,t denotes a dummy for monetary policy communication channel
w.[11] ARCH
in the residuals is addressed by including lags of the absolute value standardised residuals
|νt−x|, and lags of the logged conditional
variance terms  . Asymmetric responses to shocks can be addressed by
including lags of the standardised residuals νt−x.
Days to rollover for each futures contract are captured by the variable Other7,t.
Finally, as in the mean equation, we include time-specific dummies. Identifying the effect of
the economic commentary on days of monetary policy decisions is a particular challenge, since
there can also be a policy rate surprise on these days. We attempt to do this by controlling for
the surprise in the mean equation. Therefore, the communication dummies in the variance equation
should only reflect effects not captured by the interest rate surprises modelled in the mean
equation.[12]
. Asymmetric responses to shocks can be addressed by
including lags of the standardised residuals νt−x.
Days to rollover for each futures contract are captured by the variable Other7,t.
Finally, as in the mean equation, we include time-specific dummies. Identifying the effect of
the economic commentary on days of monetary policy decisions is a particular challenge, since
there can also be a policy rate surprise on these days. We attempt to do this by controlling for
the surprise in the mean equation. Therefore, the communication dummies in the variance equation
should only reflect effects not captured by the interest rate surprises modelled in the mean
equation.[12]
We estimate the model in Equations (3) and (6) for Australia, Canada, the euro area, New Zealand, the UK and the US, and for a panel of these economies, using fixed effects in both the mean and variance equations.[13] The equations are estimated for each of the first eight 90-day futures contracts, which measure interest rate expectations from the 3-month to 2-year horizon. We first estimated Equation (3) for each economy with all the available explanatory variables using OLS to obtain a more parsimonious model by excluding insignificant macroeconomic releases. GARCH models are estimated by the method of maximum likelihood using an iterative algorithm, since the conditional variance appears in a non-linear way in the likelihood function. We estimated the EGARCH model using a general-to-specific modelling approach, by excluding insignificant variables in a number of iterations. Similarly, we tested the appropriate dimensions of the EGARCH model for each economy separately. Interestingly, the lagged conditional variance terms in the variance equation were insignificant, except for the US, thus reducing our models to an ARCH specification. Economically, this implies that an increase in the conditional variance of interest rate futures as a result of communication does not lead to increased variance on subsequent days. Table 5 summarises the specifications and diagnostics of the final models. The overall fit of the equations are reasonable, with R-squared values of between 0.14 and 0.61.[14]
| Australia | Canada | Euro area | NZ | UK | US | Panel | |
|---|---|---|---|---|---|---|---|
| EGARCH (x,y) | (3,0) | (5,0) | (4,0) | (5,0) | (4,0) | (5,1) | (5,0) |
| Overseas effects | US | US | US | US, Aus | US | – | US |
| Diagnostics | |||||||
| R2 | 0.34 | 0.61 | 0.40 | 0.54 | 0.30 | 0.14 | 0.35 |
| ARCH LM (5) | {0.79} | {0.81} | {0.65} | {0.58} | {0.92} | {0.86} | {0.62} |
| Excess kurtosis | 2.24 | 2.25 | 0.71 | 2.88 | 1.04 | 1.59 | 1.52 |
|
Notes: Numbers in braces are p-values. Estimates for the euro area and the panel start from 1 January 1999, and for NZ from 17 March 1999. In the variance equation, x is the number of lagged standardised residuals and y is the number of lags of the logged conditional variance (see Equation (6)). |
|||||||
The variance equations for each economy include an EGARCH specification sufficient to account for any ARCH remaining in the standardised residuals. This is confirmed using ARCH LM tests. While the excess kurtosis of the interest rate futures has been greatly reduced by the EGARCH model, there is still some evidence of excess kurtosis, indicating non-normality of the standardised residuals. Therefore, Bollerslev and Wooldridge (1992) heteroskedasticity consistent standard errors are reported.[15] We now turn to specific results these estimations yielded. For brevity, we will only show the results for the 4th contract for interest rate futures in the tables, however, the figures show the results across all eight contracts. More detailed results can be found in Appendix B.
4.2 The Effect of Macroeconomic News and Monetary Policy Surprises
The results of the mean equation can tell us which macroeconomic news releases are most important for interest rate expectations. As mentioned above, we included a large number of macroeconomic surprise variables. For instance, there were 801 Australian news releases during the period, made up of 16 different types of releases, of which half significantly influenced interest rate expectations. Table 6 shows which economic releases were found to be significant in the mean equation for the change in interest rate futures (4th contract).
| Australia | Canada | Euro area | NZ | UK | US | |
|---|---|---|---|---|---|---|
| Prices | CPI | CPI | PPI (euro area) | CPI | Input PPI | CPI |
| CPI (France) | Core CPI | Output PPI | GDP deflator | |||
| Core CPI (Spain) | Input PPI | RPIX | ||||
| Labour market | Employment | Employment | Unemployment (France) | Unemployment rate | Average earnings | Average hourly earnings |
| Unemployment rate | Non-farm payrolls | |||||
| Unemployment rate | Employment cost | |||||
| Initial jobless claims | ||||||
| Activity | GDP | GDP | GDP (euro area) | GDP | GDP | Advance retail sales |
| Building approvals | Industrial production | Industrial production (euro area) | Retail sales | Industrial production | Capacity utilisation | |
| Trade balance | Manufacturing shipments | Consumer spending (France) | Consumer credit | Chicago purchasing managers' business barometer |
||
| Inventories | Retail sales excl autos | GDP (France) | Retail sales | Consumer confidence | ||
| Investment | Production outlook (France) | Trade balance | Durable goods excluding transport |
|||
| Retail sales | IFO (Germany) | Empire manufacturing | ||||
| Industrial output (Germany) | Existing home sales | |||||
| GDP (Italy) | ISM manufacturing | |||||
| GDP (Spain) | ISM non-manufacturing | |||||
| Philadelphia Fed Outlook Survey | ||||||
| Michigan confidence | ||||||
| Wholesale inventories |
For Australia, activity indicators such as retail sales, building approvals and GDP are significant along with prices and labour market indicators such as the CPI and employment. These results are consistent with those found by Campbell and Lewis (1998) and Silvapulle, Pereira and Lee (1997). While not included in Table 6, US data surprises – measured through their impact on US interest rate futures – explain a large share of movements in Australian interest rate futures. This result has been confirmed by earlier studies, such as Kim and Sheen (2000). The results for other economies are also in line with those found by previous country-specific studies, where available. For example, for the US, Kohn and Sack (2003) find that announcements of 13 economic data releases affect the Federal funds futures significantly; almost all of these are included in our list of 18 significant macroeconomic releases for the US. For Canada, Gravelle and Moessner (2001) single out surprises in the PPI, employment and US data, comparable to our results. Across economies, a number of similar releases can consistently be found to be significant. These are not surprising: CPI in the category of important price releases, unemployment in the labour market category and GDP and retail sales in the economic activity category.
The results for the mean equations can also show whether market participants view surprises in monetary policy decisions as shocks to the short-term or medium-term outlook. For Australia, interest rate futures which expire within three months (the 1st contract) respond quite strongly to monetary policy surprises, rising by around 6 basis points in response to an unexpected cash rate increase of 10 basis points (Figure 1). This response falls steadily as the settlement date becomes more distant. This suggests that market participants view monetary policy surprises as containing more short-run than medium-run information. In contrast, macroeconomic surprises such as GDP, the CPI or retail trade have a relatively consistent effect on interest rate expectations out to the two-year horizon. This suggests that they are viewed as relevant to the medium-term outlook. This is consistent with the findings of Campbell and Lewis, who report that monetary policy news has more often been associated with a large move in bill yields (that is, the short end of the futures market) while macroeconomic surprises also affected bond yields (that is, the long end of the market).
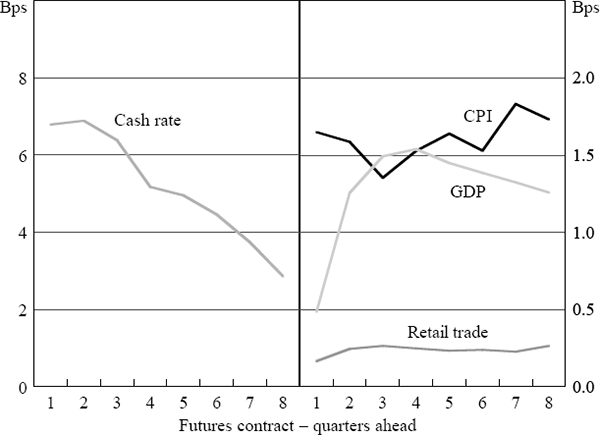
Overall, the profile for the interest rate futures response to monetary policy surprises for Australia is reasonably representative for those of the other economies, with an impact of between 5 and 8 basis points on the 1st contract, which steadily declines for the contracts further ahead. We do not report these results in more detail, since they are in line with those found by a number of other studies (see, for example, Kohn and Sack 2003 for the US, Gravelle and Moessner 2001 for Canada, and Chadha and Nolan 2001 for the UK). It is worth noting, however, that the results for New Zealand seem to have a less smooth profile, possibly because of the lower liquidity of the New Zealand futures market, especially for the longer-dated contracts.
4.3 The Effect of Monetary Policy Communication
One of the motivations of our study is to estimate the effectiveness of different channels of central bank communication, and to analyse whether we can detect consistent patterns across different economies. For this, we now turn our attention to the results from the variance equation. As stressed earlier, due to the nature of our communication variables (it is difficult to objectively measure news contained in communication events), we interpret a positive statistically significant result as ‘effective’ since it appears to have provided information to the markets.[16] We cannot, however, measure whether the information extracted by the markets is the information the central bank intended to convey.
In Table 7, the communication results from the variance equation are presented for each economy and the panel. Some types of communication, such as publishing minutes of meetings, are used only by some central banks and therefore some values are missing from this table. Other events do not occur often (such as unscheduled rate moves). We would expect such events to have a significant effect on markets precisely because they are rare. However, estimated coefficients for these events should be treated with caution since they are based on very few observations. Any coefficient based on 10 or less events is reported in braces. Again, the results are presented for the 4th futures contracts.
| Australia | Canada | Euro area | NZ | UK | US | Panel | |
|---|---|---|---|---|---|---|---|
| Commentary with rate decisions | |||||||
| Scheduled rate moves | 0.46 (0.36) |
1.09*** (0.30) |
1.42*** (0.29) |
0.32 (0.31) |
1.34*** (0.25) |
1.02*** (0.26) |
1.09*** (0.13) |
| Unscheduled rate moves | {1.87***} (0.33) |
0.76 (0.69) |
{3.76***} (0.25) |
{−18.81} (0.99) |
{1.21**} (0.53) |
1.38 (1.38) |
|
| ‘No move’ decisions | 0.25 (0.31) |
0.71*** (0.25) |
1.43*** (0.50) |
{−1.28} (0.59) |
1.18*** (0.34) |
0.93*** (0.16) |
|
| Rate decisions without commentary | |||||||
| Rate moves | {0.98***} (0.35) |
{1.28***} (0.37) |
|||||
| ‘No move’ decisions | −0.47 (0.26) |
−0.18 (0.21) |
{−1.30} (0.49) |
0.49*** (0.18) |
−1.20 (0.24) |
−0.03 (0.12) |
|
| Reports | 0.80*** (0.27) |
0.63* (0.39) |
0.00 (0.17) |
1.58*** (0.53) |
0.18 (0.22) |
1.88*** (0.21) |
0.47*** (0.12) |
| Parliamentary hearings | |||||||
| Post-reports | 1.25** (0.53) |
0.33 (0.45) |
1.40** (0.66) |
1.15* (0.68) |
1.88*** (0.21) |
1.15*** (0.29) |
|
| Other | 0.08 (0.23) |
0.59* (0.32) |
−0.08 (0.17) |
||||
| Minutes of meetings | 0.56*** (0.15) |
0.04 (0.18) |
0.37*** (0.14) |
||||
| Speeches | 0.46** (0.22) |
0.40 (0.33) |
−0.24 (0.10) |
0.18 (0.19) |
0.01 (0.12) |
0.13 (0.10) |
0.07 (0.06) |
|
Notes: Numbers in brackets are Bollerslev-Wooldridge (1992) heteroskedasticity consistent standard errors. ***, **, * indicate positive coefficients are significant at the 1, 5 and 10 per cent levels, respectively. Estimates in braces are based on 10 or less events and should therefore be treated with caution. The model for the euro area and the panel was estimated from 1 January 1999 and for NZ from 17 March 1999. The US Fed's monetary policy report and testimony occur simultaneously, so the same coefficient is reported for both. |
|||||||
Across all economies – given the size and significance of the coefficients – the most important channels of monetary policy communication are the economic commentary accompanying rate moves, parliamentary hearings and monetary policy reports; minutes of meetings and speeches are much less important. As discussed in Section 4.1, identifying the effect of the economic commentary on days of monetary policy decisions is a particular challenge, due to the concurrent policy decision. In this respect, the results in Table 7 are comforting, since policy decisions without commentary are insignificant for almost all conditional variance regressions. This suggests that the policy surprise effect is well captured by the mean equation, allowing us to identify the communication effect through the variance equation.
It is not straightforward to interpret the magnitude of the coefficients and compare them across economies, since the dependent variable of the variance equation is the logged conditional variance. To make interpretation easier, in the following figures we have transformed the coefficients in Table 7 such that they represent the average effect in basis points on the standard deviation of policy expectations for all horizons.[17] These transformed coefficients measure the change, in basis points, of the standard deviation of the interest rate futures on average on the day when a specific communication event occurs.
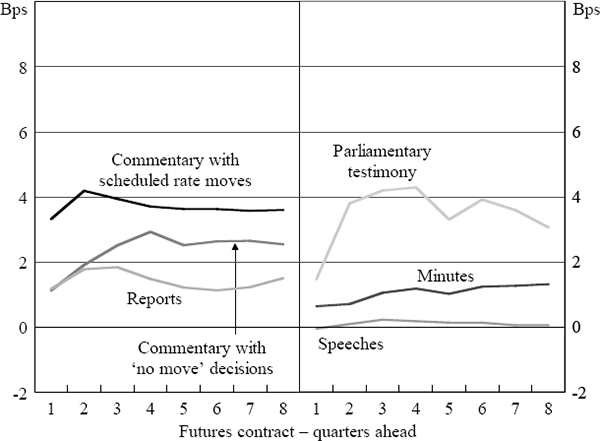
Figure 2 shows these transformed coefficients for Australia over all eight futures contracts (similar figures for the other economies can be found in Appendix B). The results indicate that parliamentary hearings have the largest impact on interest rate expectations among the various communication channels. On average, parliamentary hearings shifted the standard deviation of interest rate expectations by around 2 to 6 basis points, with the largest effect on expectations of rates in two to three quarters' time. Other channels of communication that also have an effect are the quarterly Statement on Monetary Policy (reports), the commentary accompanying scheduled rate moves and speeches. Each of these has an average effect of around 1 to 4 basis points on rates, with the largest response at the two- to three-quarter horizon.
The channels of communication which have the greatest effect on Australian interest rate expectations are also among the most important for the other central banks in our sample. We will discuss these specific results in more detail in the remainder of the section.
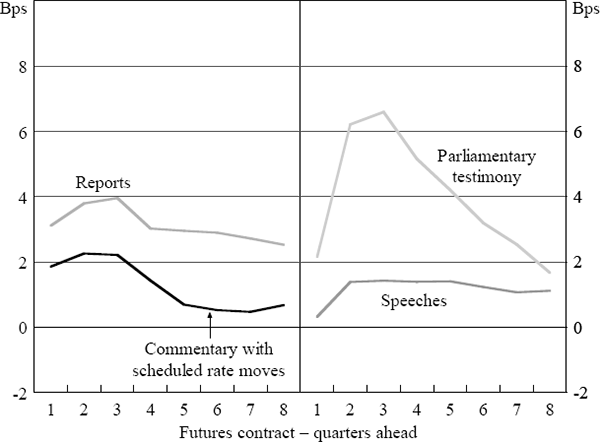
4.3.1 Commentary with monetary policy decisions
The commentary accompanying rate moves influences policy expectations significantly in all economies for the first year ahead (Table 7 and Figure 3), while ‘no move’ decisions with commentary are positive and significant in the euro area, New Zealand and the US. In contrast, ‘no move’ decisions without commentary are positive and significant only for the UK, with the panel result showing insignificance.

These results suggest that markets' interest rate expectations are influenced by the commentary accompanying rate decisions, and not just the decision itself. This is consistent with the results of Kohn and Sack (2003) for the US, and the close scrutiny given to press releases and press conferences by market participants and the media.
As noted earlier, these results cannot be used to determine whether the response of the markets was consistent with the intentions of the central bank. In the US in particular, there has been debate over whether the FOMC policy statements should be signalling future policy intentions. For instance, in August 2003, William Poole, President of the St. Louis Fed, argued that narrower language should have been used in the FOMC statement in May 2003 that warned of deflation, thereby contributing to a decline in bond rates (see Poole 2003).
4.3.2 Monetary policy reports and parliamentary hearings
Policy reports and post-report parliamentary hearings appear to be the most important channels of communication outside of rate decisions (Figures 4 and 5). The magnitude of response is largest in the US, Australia and New Zealand, where the average effect on the standard deviation of expectations is around 4–10 basis points.
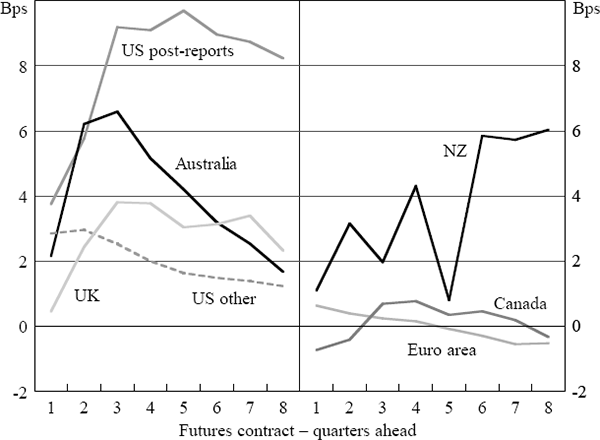
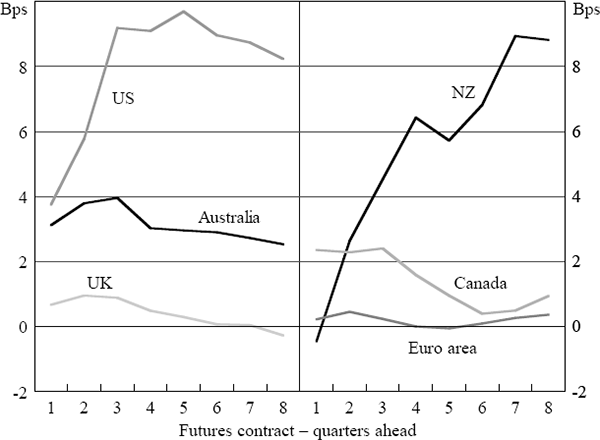
Parliamentary hearings in Australia, New Zealand, the UK and the US significantly affect the markets. Notably, the US post-report hearing, the Humphrey-Hawkins testimony, has a much larger effect than other US testimonies. Two factors are likely to play a role in this: the Humphrey-Hawkins testimony coincides with the release of the monetary policy report – so we cannot disentangle the two effects – and it occurs less frequently than the other testimonies. The results for New Zealand are also significant, but since parliamentary hearings, monetary policy reports and rate decisions often happen on the same day, the magnitudes of individual effects are harder to disentangle. Moreover, the futures market in New Zealand is less liquid than in the other economies considered here, which might explain why most of the results for New Zealand appear less ‘smooth’ across the different futures contracts.
The monetary policy reports in Australia, Canada, New Zealand and the US provide information that significantly affects the markets. For the US, this effect also incorporates any effects of the parliamentary testimony that coincides with the report, which might explain the relatively large effect. The Bank of England's Inflation Report did not significantly influence market expectations of future rates. This result is consistent with Chadha and Nolan (2001), who also found that the Inflation Report did not significantly influence interest rates. The ECB's analysis of ‘Economic and Monetary Developments’ is also insignificant. The ECB's report is published more frequently, as part of the monthly Bulletin, while most other central banks' reports are published on a quarterly or semi-annual basis. The high frequency of these reports might be one reason why the effect of an individual monetary policy report for the euro area is relatively small.
4.3.3 Minutes of meetings and voting records
Only two economies in our sample (the UK and the US) release minutes of their monetary policy committee meetings (and voting records). Only the coefficient for the UK is significant, while that for the US is insignificant and close to zero for all futures contracts. The UK minutes are significant at all horizons, in contrast with the results by Chadha and Nolan (2001) who find an insignificant effect for the UK. Given that the UK minutes are released monthly, two weeks after policy decisions, this may help to explain why the Bank of England's quarterly Inflation Report has little effect on expectations.
There are several explanations for the relative unimportance of the US minutes. First, the minutes are released with a lag of 6–8 weeks by the Fed on the day after the following FOMC meeting, which reduces their relevance for forward-looking analysis. It is also unclear whether the Fed is intending to influence expectations with the minutes. In 1997, the FOMC was concerned that the minutes were not receiving enough press, and shifted the timing of the release to try to maximise reporting in the Friday papers. The associated debate regarding the target audience of the minutes – whether it is the media, the markets or Congress – suggests that the FOMC was more concerned about how the minutes were viewed by the press and Congress than whether they are a tool to influence markets' expectations (see FOMC 1997).
These results highlight another aspect of our study. ‘Having’ a certain channel of communication is not necessarily the decisive factor. Markets will attach importance to a specific channel only if new information is conveyed. However, the same information can, in principle, be conveyed through other communication events. Consequently, the difference in results for specific communication channels across economies can often be explained by looking at the entire communication structure. For instance, minutes for the UK are released two weeks after the meeting, and are therefore likely to be one of the first communication events that convey the views of the central bank after a meeting of the Monetary Policy Committee. In contrast, the minutes of the Federal Reserve are not released until after the next meeting has taken place. In the meantime, a number of different communication events will have happened which allows the central bank to explain its views on current conditions for monetary policy.
4.3.4 Speeches
While we find that speeches have a significant positive coefficient for Australia and – at an 80 per cent significance level – also for the US, this effect is not systematic across all the central banks in our sample. One explanation could be that speeches occur relatively frequently and, therefore, information tends to be conveyed more gradually. However, this result may also be a reflection of our methodology, rather than a general statement on whether speeches are used to convey important information in these other economies. We do not subjectively choose speeches that are more likely to influence expectations. Instead, all speeches published on the websites of the central banks are included in our sample. Some of these may be speaking engagements dealing with other central bank responsibilities unrelated to monetary policy. For the economies with an insignificant overall effect, the inclusion of these speeches is likely to hide the effect of speeches that are deliberately designed to influence expectations.[18] Even if we were able to single out these speeches, we would still be left with the problem that in some instances information may be conveyed during a question and answer session following a speech.
4.3.5 Panel results
Our panel, which estimates the effect across all central banks, can provide some insights into the common factors of the communication strategies of the six central banks (Figure 6). The results suggest that across the six economies, central banks have used parliamentary hearings, commentaries with policy decisions and monetary policy reports to influence interest rate expectations. These channels of communication affect the outlook for policy in both the short and medium term. Minutes of meetings are significant, but are entirely driven by the results for the UK, while speeches do not have a significant effect.
Footnotes
For an accessible exposition of ARCH and GARCH models, see McKenzie and Brooks (1999). [9]
GARCH models of short rates often require the inclusion of the level of the interest rate in the variance equation (we would like to thank Adrian Pagan for drawing our attention to this). In our model we find that this term is insignificant (or negative) over almost all horizons for all the countries studied. One possible explanation is that this term serves to model differences in the magnitude of policy changes under high and low inflation, but for the period we studied inflation was always low. [10]
As suggested by the results in Section 3, if the communication events are included in the mean equation their average effect is insignificant. This result, however, may be due to the measurement of these variables, which does not include ‘direction’ of the information and therefore ‘upward’ and ‘downward’ movements may be netted out. Changes in the mean also affect the variance on the day of the news event, but the effect on the variance abstracts from the direction of the effect. Therefore, in our framework, the coefficient in the variance equation captures both (non-directional) changes in the mean and possible additional effects on the variance. [11]
In principle, macroeconomic and monetary policy surprises could affect both mean and variance. However, the inclusion of these variables in the variance equation yields mostly insignificant effects, suggesting that most of their effect has been absorbed by the mean equation. [12]
We estimated our GARCH model with EViews, version 3.1. The panel regression with GARCH followed the example in Grier and Cermeño (2001). [13]
A significant portion of this explanatory power comes from the ‘foreign rates’ variable, which helps to explain why the fit is lowest for the US. [14]
This approach, which uses quasi-maximum likelihood estimation, is standard in the literature; see McKenzie and Brooks (1999, p 24) and Jansen and de Haan (2003). [15]
Significant, but negative coefficients in the variance equation imply that on the day of the event the variance of the interest rate future is typically lower than on days without such events. Therefore, we are primarily interested in results when the coefficient is significantly positive. [16]
We take the average difference between the standard deviation of the errors in our regressions and those that would result if we assumed (in turn) that each channel of communication did not exist, where n is the number of communication events in the sample:
[17]Some evidence that this factor plays a role is provided by a robustness test where we decided whether a speech dealt with monetary policy based on its title. Using this split in our regressions, we find that speeches in the ‘Monetary policy’ category are significant for the euro area, the US and – for horizons up to one year – New Zealand, while those in the ‘Other’ category tend to be insignificant. [18]




