RDP 2005-12: Financial Constraints, the User Cost of Capital and Corporate Investment in Australia 2. Theory
December 2005
- Download the Paper 530KB
2.1 Definition of Financial Constraints
Under the perfect capital market assumption, the Modigliani-Miller theorem suggests that a firm's capital structure is irrelevant to its value. This implies that external finance (new debt and/or equity issues) and internal finance (retained earnings) are perfect substitutes and a firm's investment and financing decisions are independent of each other. The availability of internal funding does not matter for investment, so the price at which firms can obtain funds becomes the only financial consideration in determining the level of investment.
However, there are a number of reasons why capital markets are not perfect. In particular, taxes, transaction costs and information asymmetries (between lenders and borrowers and/or between managers and shareholders) can make external sources of finance more costly than internal finance. If markets are characterised by imperfect information, investment finance may only be available on less favourable terms in external capital markets, or may not be available at all. This implies that the investment spending of some firms may be constrained by a shortage of internal funds. As a result, the level of internal funding could be an important determinant of investment empirically. To understand this concept of financial constraints it is useful to consider Figure 1.[2]
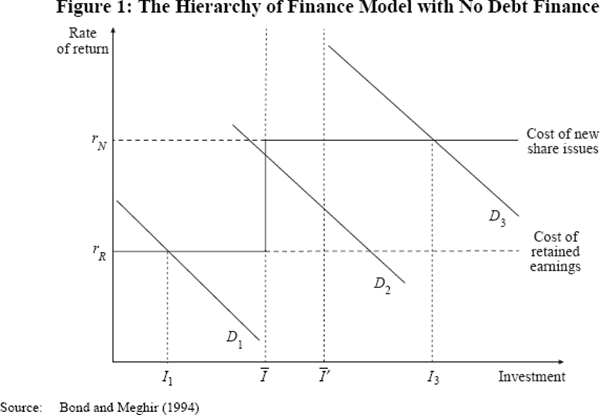
The rate of return rR shows the cost of financing investment out of retained earnings (that is, the required rate of return on these funds). The (higher) rate of return rN shows the cost of finance from say, new share issues. The downward-sloping schedules (D1, D2 and D3) illustrate three possible sets of investment opportunities available to a given firm, relating the required rate of return to the feasible level of investment.
Assume that the level of investment spending, Ī, is the maximum level of investment that the firm can finance with internally available funds. If the firm has relatively limited investment opportunities (that is, the firm is on a curve like D1) then the firm can finance all of its desired investment from retained earnings. The firm's investment level would occur at I1 and would not be affected by minor fluctuations in cash flow around the level corresponding to the maximum level of investment, Ī. If the firm has relatively large investment opportunities (that is, it is on a curve like D3) then it may find it worthwhile to make use of external finance, despite the higher costs of such funding. Their investment would be at the level I3, which, again, is unaffected by minor fluctuations in cash flow around the level corresponding to Ī.
Consider the intermediate case, illustrated by the curve D2, where financial constraints (in the form of limited retained earnings) affect the investment spending of firms. Such firms have sufficiently profitable investment opportunities that they exhaust all their internal funds. However, their remaining projects are not so attractive that they can cover the higher rate of return required by external funding sources. The investment spending of such constrained firms is limited to the level that can be financed from retained earnings.
A ‘financially constrained’ firm can be thought of as a firm whose investment spending would rise (fall) if its retained earnings increased (decreased). For example, a rise in retained earnings would shift the maximum level of investment that can be financed internally, say from Ī to Ī′, so that there would be a commensurate increase in the investment of constrained firms.
Including debt finance complicates the story but the implications for investment remain basically the same.[3] If the cost of debt finance rises with the amount raised (commensurate with the higher risk of default), the supply schedule for external funds has a kink in it at the investment level, Ī (Figure 2). For firms in Regimes 1 and 3 the situation is basically unchanged, although Regime 3 firms can now finance investment through both debt and equity issues.
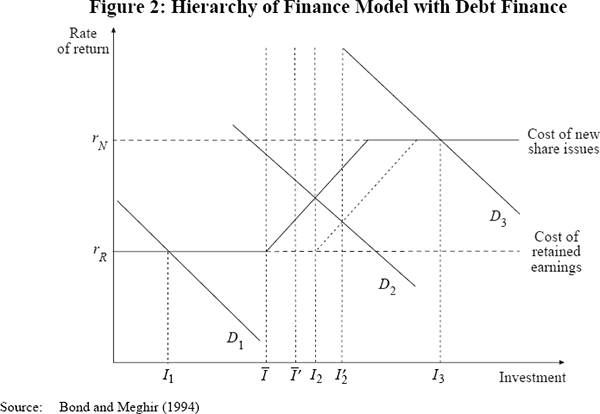
On the other hand, firms in Regime 2 are no longer constrained to the level of investment given by Ī. They can finance higher investment by borrowing to the extent that they find it worthwhile to bear the increasing cost. In this case, their investment is determined by the rising cost of debt, giving the level I2. However, they are still financially constrained since an increase in cash flow would allow levels of investment above Ī to be financed at lower levels of borrowing. This reduces the effective cost of debt at each level of investment, resulting in higher investment at I2′. As before, the investment of firms in this position is limited by the availability of internal finance, even though they have access to external finance.
Some of the channels of monetary transmission can also be distinguished using Figure 2. Take, for instance, the case of a tightening of monetary policy. This will increase the required rate of return on retained earnings, rR, and shift up the supply schedule for external funds. This rise in the relative cost of capital will reduce the investment of all firms, regardless of whether they are constrained or not. This effect is commonly referred to as the interest-rate channel of monetary policy.
However, the rate rise will also increase the gradient of the loan supply schedule. This happens either because the rate rise increases the net interest payments (reduces the cash flow) of leveraged firms, or because it reduces the discounted value of assets used as collateral.[4] In either case, the firm's risk premium (and hence effective cost of borrowing) should rise. This, in turn, will restrict the amount of funding available, thereby affecting the investment behaviour of financially constrained firms – the credit channel of monetary policy.
This theory implies that it may be necessary to include a measure of internal funding (such as cash flow) in an estimated model of investment spending. However, it is important to note that finding a significant role for internal funding measures in explaining investment does not necessarily imply that some firms are financially constrained. If the estimated model does not adequately control for investment opportunities then internal funding could be significant because it provides information about future profitability and hence investment. A structural Q-type model may alleviate this problem and this model is discussed in Section 4.[5]
2.2 Measurement of Financial Constraints
To assess the effects of financial constraints on corporate investment requires financially constrained firms to be identified. Unfortunately, there is no consensus as to the best method of measuring (unobservable) financial constraints. Studies have tried to identify financially constrained firms on the basis of dividend payout ratios, firm size, age, industrial group membership, the nature of the bank-firm relationship, the presence of bond ratings and the degree of ownership concentration (Schiantarelli 1996).
This paper uses reductions in dividend payments as an indication of financial constraint (Cleary 1999; Siegfried 2000). Firms that are unconstrained have no incentive to cut dividend payments while constrained firms can cut dividends to free up internal funds for profitable investment projects. Therefore, firms are classified as financially constrained if they have cut nominal dividend payments in either the current or previous period. This has the advantage over more ad hoc identification schemes since the decision to cut dividends is part of the firm's optimisation problem (Schiantarelli 1996). By using a dummy for periods in which a firm makes a dividend cut, this classification scheme also allows firms to switch between constrained and unconstrained ‘regimes’ over the sample period.
However, it is important to recognise that a firm's dividend policy may not always be a good indicator of the level of constraint. In particular, in recent years, firms in certain industries have tended to move away from paying dividends. This is likely to dilute the impact of dividend changes on the level of retained earnings and hence, potentially, investment spending. For instance, in the sample of firms used in this paper, over 75 per cent of firms in the biotechnology, energy and telecommunications industries do not pay (or report) dividends (Figure 3).
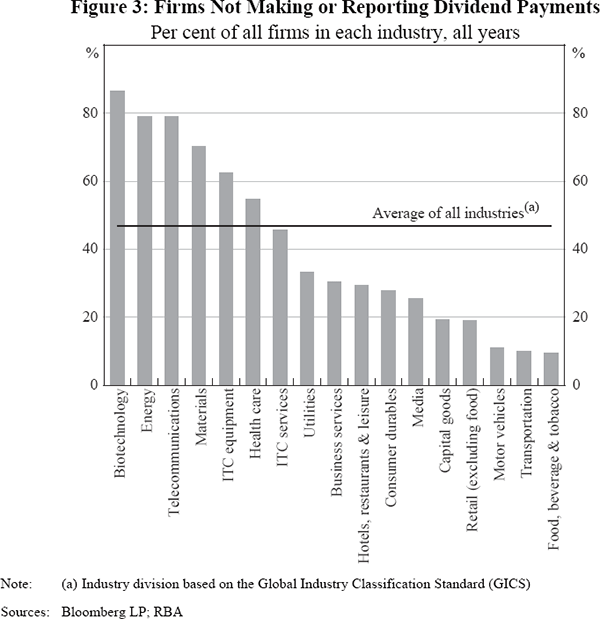
Econometric analysis nonetheless suggests that dividend cutting is a useful measure of financial constraints in our data sample.[6] One indication of this is that the proportion of firms identified as being financially constrained appears to be counter-cyclical, which seems fairly intuitive. I also experimented with other measures of financial constraints, including dividend payout ratios, firm size and age, the presence of corporate bond ratings and the firm's implicit risk premium, measured as the difference between a firm's average interest rate on debt and the risk-free interest rate (proxied by the yield on 10-year government bonds). The results of these exercises were qualitatively similar to those presented in this paper.
2.3 Financially Constrained versus Financially Distressed Firms
Assuming financial constraints are measured correctly, the preceding discussion implies that the investment of constrained firms should be more responsive to a given change in cash flow than the investment of unconstrained firms. However, a number of studies have found that the difference between the two groups is insignificant or that the investment of unconstrained firms is actually more responsive (Gilchrist and Himmelberg 1995; Kaplan and Zingales 1997; Cleary 1999; Allayannis and Mozumdar 2004).
One reason that constrained firms may not display a higher sensitivity to cash flow is because the sample of constrained firms could include firms that are in financial distress (Fazzari, Hubbard and Petersen 2000). Financially distressed firms can be thought of as those with negative cash flow. Such firms may be in such difficulty that they can only make absolutely essential investments so that any changes in investment in response to cash flow could be very limited. Even if their cash flow were to rise significantly, financially distressed firms have incentives to use the additional cash to reduce their debt levels, rather than using it for investment. As a result, the sensitivity of corporate investment to cash flow could be very low for these firms.[7] If there are enough distressed firms in the sample, they may dampen the effect of cash flow on investment in an estimated model.
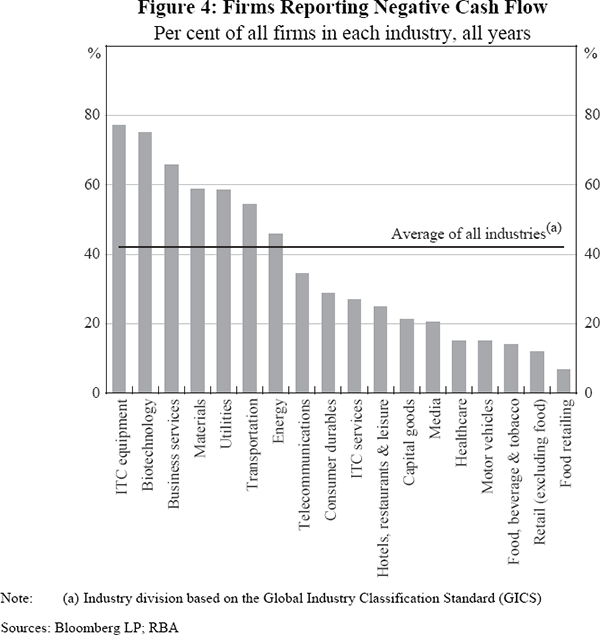
Empirically, such concerns also appear reasonable when considering the firms in the industries that account for most of the negative cash flow observations – generally firms in the ITC and biotechnology industries (Figure 4).[8] These industries are characterised by firms that are generally young and growing rapidly, but which also face large start-up costs. For these firms, cash flow can be low (and falling) and the risk of default high even when prospects for future sales are good and the firms are investing heavily. Overall, these firms comprise over two-fifths of the sample and so will need to be accounted for in the estimated model.[9]
Footnotes
The discussion and graphical analysis are adopted from Bond and Meghir (1994). [2]
Debt finance is typically less costly than equity finance since debt holders will have priority over equity holders in the event that the firm goes bankrupt. [3]
The rate rise will only directly affect the cash flow of leveraged firms with outstanding floating-rate debt. The example also does not consider that firms may hedge against interest rate risk, which could partly dilute the effect of the credit channel on investment. [4]
However, there remains the possibility that internal funding is significant because managers use free cash flow (cash flow remaining after investment in profitable projects has been realised) to over-invest. The ‘free cash flow hypothesis’ suggests that if managers engage in such sub-optimal investment policies, the Q model is also not an appropriate description of firm investment behaviour (Jensen 1986). While this remains a possibility, free cash flow is difficult to observe and testing this hypothesis is beyond the scope of this paper. [5]
In particular, I estimated a simple fixed-effects panel regression, regressing profitability (the log of cash flow/assets) on year-fixed effects, industry dummies and a variable indicating dividend cuts (DIVCUT) under the hypothesis that the coefficient on DIVCUT should be negative and significant. The year-fixed effects should partly control for business cycle effects while the industry dummies should account for structural differences in dividend policies across sectors. The fact that I find a negative coefficient on the dividend cutting variable, which is significant at the 1 per cent level, implies that our measure of financial constraints is a reasonable proxy. [6]
Another possible concern is the assumption of a positive (linear) relationship between changes in cash flow and changes in investment. Some recent studies have argued that while changes in investment may be positively correlated with cash flow for certain levels of internal funding, at other levels, changes in investment may be negatively correlated with cash flow. In other words, a firm's optimal investment function could be hump-shaped over a range of internal funding levels (Povel and Raith 2001; Allayannis and Mozumdar 2004; Cleary, Povel and Raith 2004; Cunningham 2004; Moyen 2004). However, I do not find compelling evidence for the hump-shaped hypothesis, which requires the investment of constrained firms to be sensitive to cash flow but also the investment of unconstrained and distressed firms to be relatively insensitive. [7]
The measure of cash flow used in this paper is net profits after tax plus depreciation. If firms have engaged in new investment during the year, this will effectively reduce measured cash flow in that year. This is the standard measure used in the literature (for example, Von Kalckreuth 2001). [8]
The overall proportion of firms reporting negative cash flow is much lower (though still quite high) in the final sample used in the regressions. This is because the exclusion criteria remove many of these firms from the final estimation (see Section 3.1). [9]