RDP 9304: Exchange Rate Pass-Through: The Different Responses of Importers and Exporters 5. Estimating the Pass-Through Relationship
May 1993
- Download the Paper 115KB
5.1 Econometric Method
Valid estimation requires that account be taken of the time series properties of the data to be employed. All data to be used in the estimation of the pass-through relationship are non-stationary.[20] When data are non-stationary, conventional estimation may lead to spurious regression results (Granger and Newbold 1974; Phillips 1986). An approach to dealing with non-stationary data which is increasingly employed in econometric estimation combines the concepts of cointegration and error correction.
In essence, if two series are non-stationary, but a linear combination of them is a stationary process, they are said to be cointegrated (Engle and Granger 1987). In this case, standard regression analysis can be usefully employed. Importantly, cointegration not only facilitates valid estimation, it also provides a framework with which to model the long-run equilibrium of an economic system. Furthermore, the presence of a cointegrating relationship implies the existence of an error-correction equation which can describe the short-run dynamics of adjustment towards equilibrium. Economic theory provides strong priors about the existence and possible nature of long-run relationships, but little information about the dynamics of adjustment. Thus the technique of cointegration can provide useful insights into the mechanics of reversion to a steady state. This is especially pertinent to exploring the process of exchange rate pass-through.
Most research on the Australian pass-through relationship was conducted prior to the convention of testing for stationarity. Important exceptions include the work by Menon (1991b, 1991c) and Heath (1991) where cointegration techniques were employed.[21] In this paper, the cointegrating relationship between the exchange rate and domestic prices is first tested and estimated using the Phillips and Hansen (1990) fully modified OLS estimator (P-H). To confirm that these results are robust, corresponding results from an unrestricted error correction model (UECM) are provided in Appendices 3 and 4. The two models produce broadly consistent results.
5.2 Import Price Pass-Through
(a) The Long-Run Relationship
To estimate the long-run pass-through relationship for import prices, a log linear form of equation (1) is used which allows for a constant so that:
The estimation period is from the September quarter 1974 to the December quarter 1992. Results from the P-H estimator confirm the existence of a cointegrating relationship between import prices, world prices and the import weighted TWI. The long-run elasticity estimates are shown in Table 1.
| Long-Run Elasticities | ||
|---|---|---|
| TWIm | WPm | |
| IPDm | 0.93 | 0.96 |
| standard errors | 0.03 | 0.04 |
The exchange rate elasticity, whilst very high, is significantly different from unity at the 5 per cent significance level. It is not different from unity, however, at the 1 per cent level. Similarly, the world price elasticity is insignificantly different from unity at the 5 per cent level.[22] Thus, with respect to the long run, these results satisfy priors about Australia as a small open economy which is a price taker for imports so that exchange rate pass-through is complete (or at least very near complete).
(b) The Short-Run Dynamics
The residuals from the P-H estimation represent the deviations from long-run equilibrium. These are substituted into an error correction model (ECM) in order to analyse the dynamics of adjustment towards long-run equilibrium. The ECM specifies changes in import prices as a function of lagged residuals from the cointegrating regression (hence, error correction), and changes in other explanatory variables. Details of the lag structure adopted and diagnostics are presented in Appendix 4.
If adjustment to an exchange rate shock were instantaneous, import prices would follow their long-run equilibrium precisely. Figure 5 shows that import prices deviate from their long-run equilibrium for periods, on average, of about one year. This observation is confirmed by the impact elasticities derived from the estimated ECM. In Figure 6, the exchange rate impact elasticity shows the estimated dynamic response of import prices to a 1 per cent depreciation. Import prices are estimated to rise by around 0.5 per cent in the same quarter in which the depreciation occurs. After one quarter, import prices have risen by almost 0.8 per cent. It takes about one year before the depreciation is completely passed through. (Pass-through of world prices is complete after three quarters.)

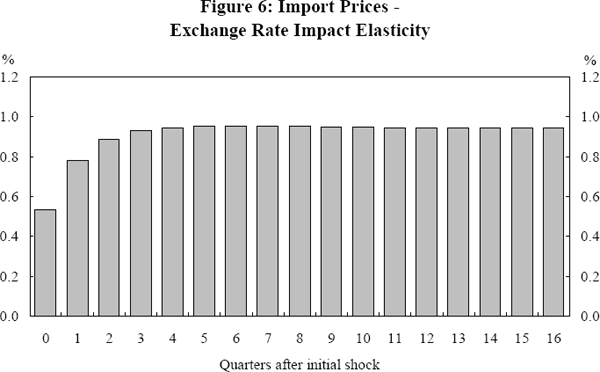
Whilst impact elasticities are useful for the purpose of generalisations about the price effects of depreciation, they cannot provide information about changes in the pass-through relationship over time. In this paper, recursive estimation techniques are used to assess whether or not the pass-through relationship has altered with respect to the exchange rate.
The stability of the path of adjustment is tested by recursive estimation of the P-H ECM (in which the long-run equilibrium is fixed to that estimated using the full sample period). The P-H ECM is re-estimated up to the September quarter 1984 and then successively re-estimated by extending the sample period one quarter. The effect of a 1 per cent depreciation is analysed. The results are illustrated in Figure 7. Each line traces the extent to which pass-through is estimated to have occurred by the quarter shown. Moving along each line shows how the path of adjustment alters with the extension of the sample. Clearly, the path of adjustment towards long-run equilibrium has remained remarkably stable.[23]
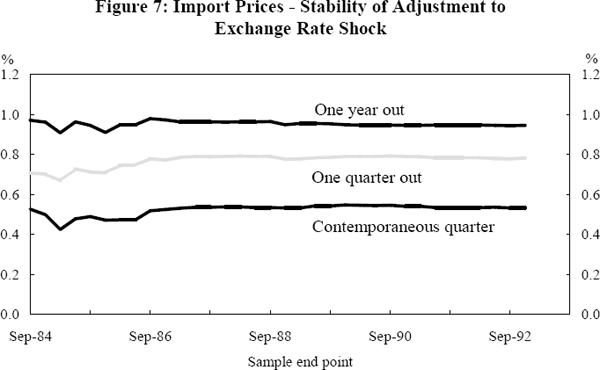
Such stability of the pass-through relationship is reinforced by out of sample forecasts. The P-H ECM is re-estimated over the period up to the September quarter 1991. It is then used to simulate import prices over the year to the December quarter 1992. Over this period, the TWIm depreciated by 13.4 per cent. If this has been fully passed through, import prices would have risen by about 14.6 per cent in line with their long-run equilibrium. The out of sample forecast using the P-H ECM implies that import prices should have risen by 11.8 per cent to the December quarter 1992. Actual import prices have risen by 10.9 per cent. The current rise in import prices is, therefore, broadly consistent with historical experience.
5.3 Export Price Pass-Through
(a) The Long-Run Relationship
Similarly, to estimate the pass-through relationship for exports, a log linear form of equation (1) is used which allows for a constant so that:
However, contrary to the case for imports, the evidence of a cointegrating relationship is not as clear. Unit root tests from the residuals from the P-H estimation cannot reject the null hypothesis of no cointegration. Nevertheless, the coefficient on the error correction term is significant for both the P-H ECM and the UECM. This represents evidence of cointegration, as discussed in Appendix 3.
The paper proceeds on the basis that a cointegrating relationship does exist. First, theory suggests that there exists a long-run relationship between changes in the exchange rate and changes in domestic export prices. Furthermore, for a small country like Australia it is expected that pass-through will be complete in the long run. Second, inspection of the data reveals that manufactured export prices tend to deviate from their long-run equilibrium for longer periods than do import prices. This does not imply that there is no long-run relationship, but it does make it more difficult to establish a cointegrating relationship when the sample period is short, as in this case. Evidence in support of a cointegrating relationship is given in Appendix 3. The long-run elasticity estimates for manufactured export prices are shown in Table 2. As for imports, they are derived from the P-H estimators.[24]
| Long-Run Export Price Elasticies | ||
|---|---|---|
| TWIx | WPx | |
| IPDx | 1.09 | 0.90 |
| standard errors | 0.12 | 0.09 |
These elasticities are insignificantly different from unity. They show that, in the long run, changes in the exchange rate will be completely passed through to manufactured export prices. Similarly, in the long run, changes in world prices are completely passed through.
(b) The Short-Run Dynamics
An ECM was estimated using residuals of the P-H estimator. (Features of the ECM and its diagnostics are presented in Appendix 4.)
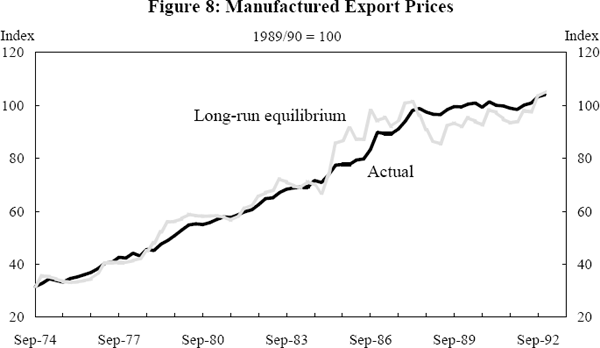
Again, if the adjustment process were instantaneous, export prices would follow long-run equilibrium precisely. Figure 8 shows that manufactured export prices deviate from their long-run equilibrium for periods, on average, between two and three years; significantly longer than is the case for imports. As shown in Figure 9, following a 1 per cent depreciation, export prices are estimated to rise by around 0.3 per cent in the same quarter in which the depreciation occurs. After one year, export prices have risen by only about 0.65 per cent, and it takes two years before most of the depreciation is passed through. (For world prices, pass-through is estimated to be near complete in the contemporaneous quarter.)
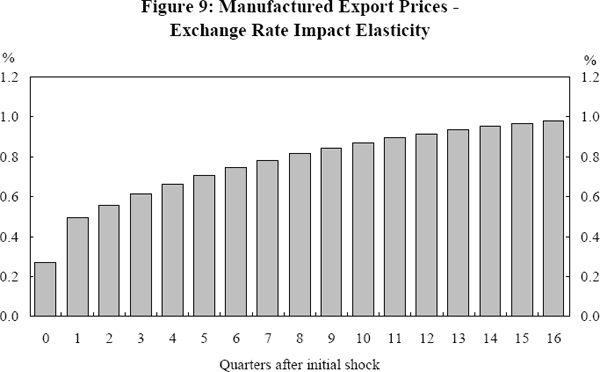
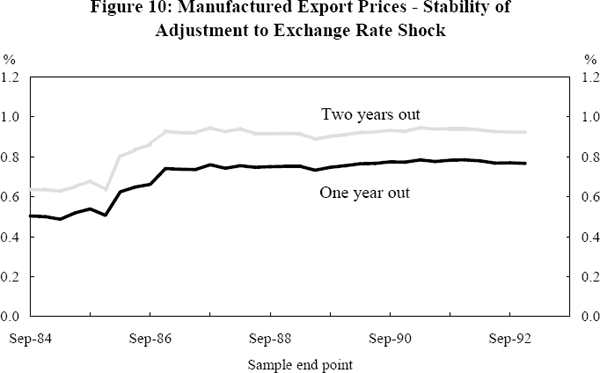
Recursive estimation is also conducted for exports to assess the stability of the pass-through relationship over time, with respect to the exchange rate.[25] Results indicate a structural break, with exchange rate pass-through becoming more rapid after 1986/87. The existence of the break is confirmed by the results of rolling regressions. The results of rolling regressions suggest that the current speed of pass-through is slightly greater than those based on the full sample period. The rolling regression results are illustrated in Figure 10, which shows the estimated effect of a 1 per cent depreciation. They indicate that up to the December quarter 1985, pass-through of the exchange rate is estimated to be only about 50 per cent complete after one year, and 60 per cent complete after two years. Conversely, for each sample period ending after the September quarter 1987, pass-through of the exchange rate is, on average, about 80 per cent complete after one year and 90 per cent complete after and two years. This suggests that since the mid 1980s, pass-through of the exchange rate to manufactured export prices has become more rapid.[26]
Footnotes
Details of the unit root tests are provided in Appendix 1. [20]
Menon (1991b) was the first in the Australian pass-through literature to address the issue of non-stationarity by employing the Engle-Granger procedure to estimate a cointegrating relationship. Menon (1991c, 1992b) also tested for cointegration using the Johansen procedure. Previous studies had implicitly assumed that regressors were stationary or subject to a deterministic trend. For example, Phillips (1988) and Coppel, Simes and Horn (1987) estimated with log-levels, while Richards and Stevens (1987) used percentage changes. Lattimore (1989) performed a variety of estimation techniques and concluded that estimation with log levels minimised bias. [21]
Note, however, that the UECM estimates suggest a slightly lower long-run elasticity for world prices. (See Appendix 3.) This would suggest that for world prices, pass-through is near complete rather than complete. A similar pattern is found for manufactured export prices and world prices. This could also reflect the fact that the world price index is an imperfect proxy. [22]
A recursive estimation of the Bewley (1979) transformation of the UECM was conducted to ensure that the long-run equilibrium was itself unchanged. This generated long-run elasticities for each successive sample period. From this it could be concluded that the long-run relationship was also stable. Stability of the path of adjustment was confirmed by the results of a rolling regression (for which the sample length is held fixed, with the first quarter being removed from the sample as each new quarter is added). [23]
With corresponding elasticities derived from the UECM shown in Appendix 4. [24]
Again, the model is re-estimated up to the September quarter 1984 and then successively re-estimated by extending the sample period by one quarter. [25]
However, it is not possible to draw conclusions regarding whether the long-run relationship has changed over time. Results from the recursive estimation of the Bewley transformation of the UECM fail to provide conclusive evidence of stability of the long-run equilibrium. Given that manufactured export prices deviate from their long-run equilibrium for extended periods, it is not feasible to estimate the long-run equilibrium over shorter sample periods. [26]

