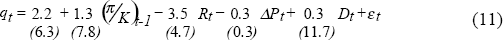RDP 9610: Share Prices and Investment 4. Share Prices And Investment – Empirical Results
December 1996
- Download the Paper 121KB
The previous section established that there was evidence of short-run inefficiencies in share prices. In this section we examine the relationship between share prices and investment and, to the extent that share-market inefficiencies do exist, we test the degree to which investment decisions are influenced by speculative movements in share prices.
Three empirical approaches are undertaken to investigate the theoretical linkage between share prices and investment. The first approach employs vector autoregressions to examine whether share prices explain investment after controlling for other variables thought to be important determinants of investment. The second approach more formally estimates investment equations and examines the importance of share prices in such equations. The final approach decomposes share prices into estimates of their fundamental and speculative components which are then incorporated into the preferred investment equation.
Briefly, we find weak evidence of a relationship between share prices and investment although once share prices are decomposed into fundamental and speculative components, the relationship between fundamental share prices and investment is much stronger. Once other determinants of investment are added to the investment equation, however, the fundamental share-price variable becomes insignificant. No relationship is found between speculative share-price movements and investment.
4.1 Causality Tests
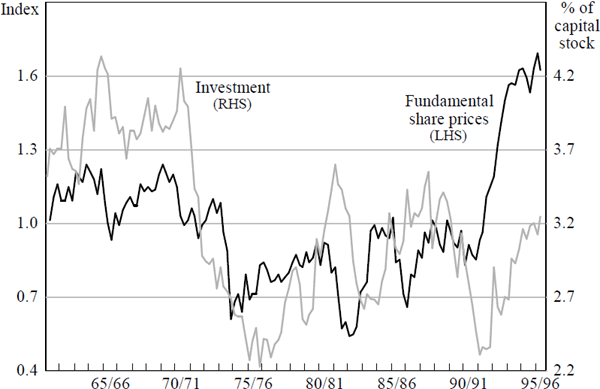
The ‘q’ theory of investment says that if q is greater than one, the market value of the firm exceeds its replacement cost and it is in management's interest to invest. A casual inspection of the data suggests that real share prices, our proxy for ‘q’, appear to be stationary (Figure 3)[15] and that there are a number of episodes where they have led movements in corporate investment (Figure 4).
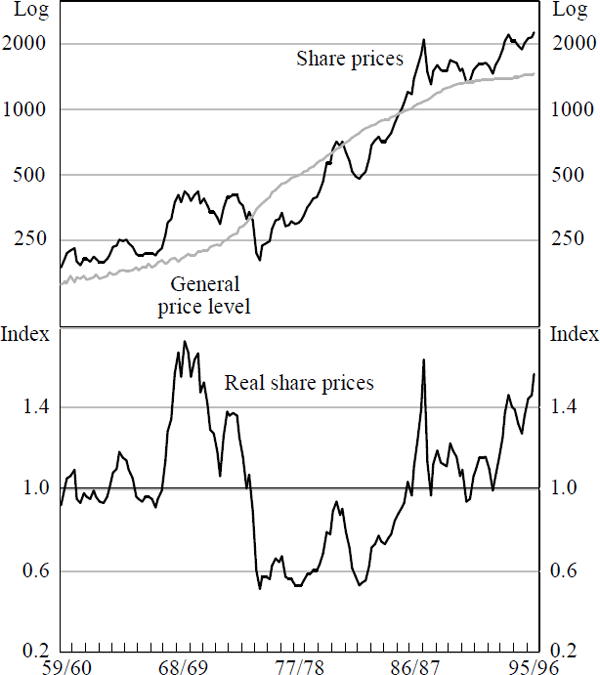
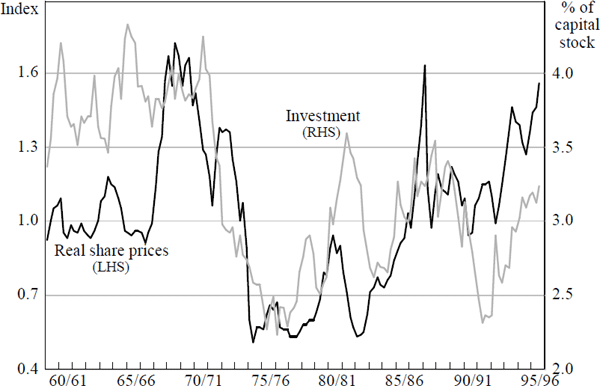
This impression tends to be confirmed in two-variable vector autoregressions (VARs) of the log of real share prices and corporate investment (as a ratio to the capital stock). Granger-causality tests indicate that, at the five per cent level of significance, real share prices do provide significant leading information on investment (Table 3).
| Variables in system |
Quarterly 1962:Q1–1996:Q1 |
|
|---|---|---|
| Two-variable VAR | Investment, share prices | 2.6* |
| Four-variable VAR | Investment, share prices, real cost of capital, real return on capital | 0.9 |
Notes: The figures are F-statistics from testing the null hypothesis that the lags of the coefficients of real share prices are jointly equal to zero. * indicates the coefficient is significantly different from zero at the 5% significance level. The VARs include four lags of each variable. |
||
It would be premature, however, to conclude from this two-variable VAR analysis that share prices cause investment. VARs rely on timing to identify causation. To the extent that share prices reflect market expectations of the future earnings of a firm, share prices are forward-looking variables. As such, the apparent leading property of real share prices may simply reflect the fact that they incorporate expected movements in variables which are also important determinants of investment. Thus, it is doubtful that a two-variable VAR can be used to decisively determine the issue of whether real share prices are exogenous and ‘cause’ investment.
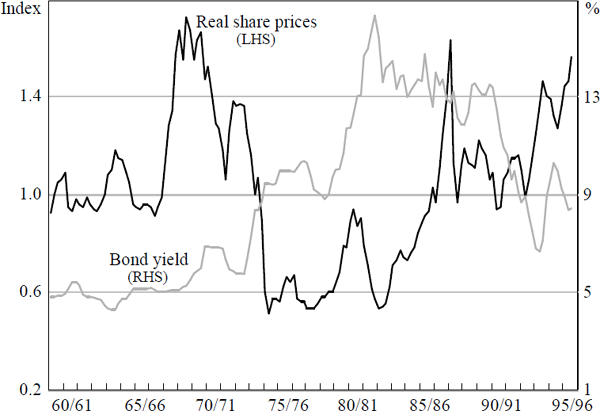
In an attempt to resolve this issue, the two-variable VAR is extended and re-estimated with variables thought to be important determinants of investment. A four-variable VAR incorporating the real cost of capital (proxied by the real 10-year bond yield) and real return on capital is estimated. It shows that real share prices are no longer significant (Table 3). These results tend to suggest that share prices are only a passive predictor of investment.[16]
Similar empirical studies conducted overseas present conflicting results. Morck et al. (1990) conclude that share prices are only a passive predictor of investment while Fischer and Merton (1984) and Doan et al. (1983) conclude that share prices are causal determinants of investment. Further, Mullins and Wadhwani (1989) found that their results varied between countries; in Japan and Germany, share prices provided no additional explanatory power for investment behaviour whereas they significantly affected investment in the UK and US.
4.2 Investment Equations with Aggregate Share Prices
The implied causal relationship presented above is tested more formally in this section. We begin by specifying an investment equation (Equation (9)) whereby investment is estimated by lags of itself and real share prices (q). We begin with a general equation with four lags of each explanatory variable and eliminate those which are insignificant to arrive at our preferred equation. The results of this estimation are shown in Table 4. At the five per cent level of significance, real share prices seem to have a role in explaining investment. As with the simple two-variable VAR approach, however, this result is relatively inconclusive as share prices may be insignificant once other variables which explain investment are included. To test this, we incorporate a vector of variables (Z) traditionally regarded as important determinants of investment (Equation (10)).
| Quarterly 1962:Q1–1996:Q1 |
||
|---|---|---|
| Explanatory variables | (9) | (10) |
| constant | −0.30** (2.7) |
−0.25* (2.3) |
| (I/K)t−1 | 0.91** (29.0) |
0.85** (23.2) |
| (π/K)t | – | 0.09** (3.3) |
| output gapt | – | 0.99** (3.4) |
| Δ Pt−1 | – | −0.44* (2.1) |
| qt−2 | 0.03* (2.0) |
0.01 (0.6) |
| Summary statistics: | ||
 |
0.90 | 0.91 |
 |
0.06 | 0.05 |
| Serial correlation test | 0.16 | 0.57 |
Notes: * and ** indicate the coefficients are significantly different
from zero at the 5% and 1% significance levels. The figures in parentheses are
t-statistics. ‘ |
||
The results from estimating Equation (10) are also presented in Table 4. Incorporating a general to specific methodology, we found that a number of variables including the real cost of capital, company sales and a measure of business confidence were insignificant and were subsequently dropped from the equation.[17] The significant variables which were retained included the real return on capital, the output gap (a measure of capacity utilisation) and inflation. The real return on capital, the output gap and the first lag of investment are all correctly signed and highly significant at the one per cent level, while inflation is also an important determinant of investment at the five per cent significance level.[18] Real share prices, however, become insignificant.
4.3 Investment Equations with Fundamental Share Prices
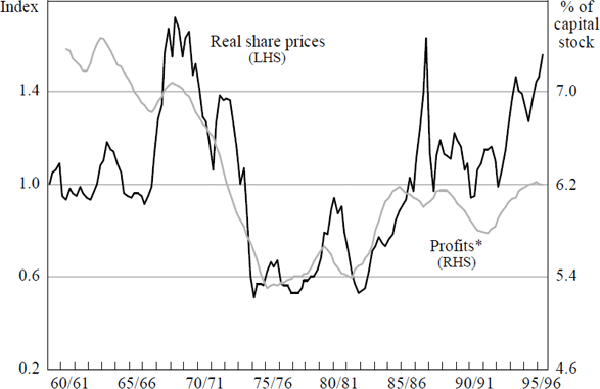
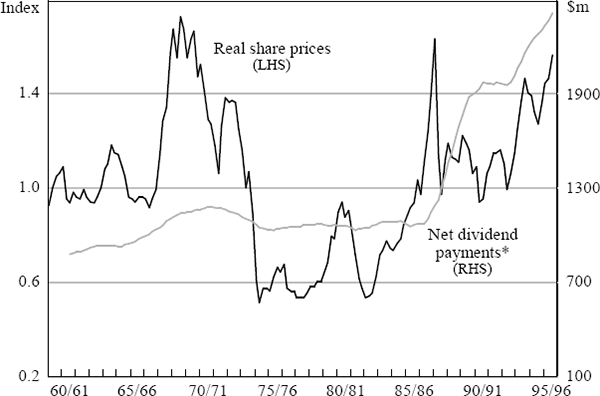
It would be premature, however, to discount the Tobin's ‘q’ relationship. In Section 2 (Equation (6)) we showed that speculative factors can distort the market valuation of a firm and that this, in turn, can obscure the relationship between share prices and investment. In order to test the validity of this proposition we need to first separately identify the speculative and fundamental components of real share prices. As indicated in Section 2, the fundamental value of a firm is the discounted present value of the firm's expected cash flows. Thus, at a very basic level, the principal determinants of real share prices are future profits and a discount rate. The relationship between these series and real share prices is illustrated in Figures 5 and 6. Implicitly, the expected relationship between share prices and profits is well established with market analysts who often focus on company price-to-earnings ratios (Figure 7).
Note: * Three-year centered moving average.

Although there is a clear long run relationship between company profits and real share prices, it appears to break down somewhat from the middle of the 1980s. This period closely coincides with the introduction of dividend imputation (a means by which the double taxation of dividend income is minimised). It is evident from the relative stability in the dividend payments series that investors have a preference for steady income streams. As a consequence, managers would tend to maintain dividend payments which are consistent with the long-term fundamental value of the firm. While imputation has clear benefits for investors, it also increases the fundamental value of the firm by reducing the cost of equity finance. As such, although the increase in net dividend payments following the introduction of dividend imputation indicates a change in investor preference to dividend income (Figure 8), it could also be seen as indicating a permanent increase in the fundamental value of the Australian corporate sector.
Note: * Three-year centred moving average.
With these observations in mind, we estimate a real share-price equation where the real share price is explained by the real return on capital, a discount rate, and net dividends paid (which captures the effect of the introduction of dividend imputation in September 1987). Rather than measuring the discount rate with the real bond yield, the nominal bond yield and inflation are entered on the basis that economic agents are slow to adjust to a new inflationary environment and, as such, inflation could reasonably be expected to be another important determinant of real share prices, at least in the short run. The specification of our parsimonious real share-price equation is presented in Equation (11) below:
 =0.53 =0.53
|
SE = 0.22 |
| sample period : 1962:Q1–1996:Q1 | |
The equation fits the data reasonably well.[19] The return on capital, the discount rate and net dividend payments are all highly significant and correctly signed. Inflation is insignificant, but nonetheless was retained in the equation since it is correctly (negatively) signed. Using this equation we are able to separately identify estimates of the fundamental and speculative components of share price movements; the fitted values representing the fundamental component (qF) and the residuals representing the speculative component (qS). Figure 9 shows that the fundamental real share-price series is less affected by the events leading up to and including October 1987.

A casual inspection of the data suggests a significant and leading relationship between fundamental share prices and investment (Figure 10). In order to validate this observation and to test the hypothesis that managers ignore speculative movements when formulating investment decisions, we examine the significance of the coefficients on the fundamental, speculative and aggregate share-price series in an investment equation. Equations (9) and (10) are re-estimated with the fundamental and speculative real share-price series replacing the aggregate real share-price series (Table 5). In contrast to the aggregate series, the fundamental series is highly significant in the simple investment equation (Equation (9a)). However, once other important determinants of investment are included it becomes insignificant (Equation (10a)). The speculative series is insignificant when included in either of the investment equations.
| Quarterly 1962:Q1–1996:Q1 |
||
|---|---|---|
| Explanatory variables | (9a) | (10a) |
| constant | −0.31** (2.7) |
−0.26* (2.4) |
| (I/K)t−1 | 0.91** (27.3) |
0.85** (23.0) |
| (π/K)t | – | 0.08** (2.9) |
| output gapt | – | 1.02** (3.4) |
| ΔPt−1 | – | −0.44* (2.2) |
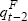 |
0.06** (3.4) |
0.01 (0.6) |
 |
0.01 (0.2) |
0.01 (0.4) |
| Summary statistics: | ||
 |
0.90 | 0.91 |
 |
0.05 | 0.05 |
| Serial correlation test | 0.40 | 0.61 |
Notes: * and ** indicate the coefficients are significantly different
from zero at the 5% and 1% significance levels. The figures in parentheses are
t-statistics. ‘ ’ is the standard error of
the equation and ‘serial correlation test’ is the Lagrangian multiplier
test for up to fourth order serial correlation with the marginal significance shown. ’ is the standard error of
the equation and ‘serial correlation test’ is the Lagrangian multiplier
test for up to fourth order serial correlation with the marginal significance shown.
|
||
It is well established in the Tobin's ‘q’ literature that ‘running horseraces between competing investment equations has shown that q-models are outpaced by equations including cash flow, output, and other flow measures of corporate activity’ (comments by Poterba in Morck et al. 1990, p. 209). In this section, we have established a similar conclusion based on Australian data. However, our results go further by addressing the question of whether managers are sufficiently sophisticated to distinguish between the fundamental and speculative movements in share prices and respond only to fundamentals. We find that investment is influenced by the fundamental level of real share prices but is not significantly influenced by the speculative component.[20] These results are broadly similar to overseas studies discussed above.
Footnotes
The unit root test results in Appendix A indicate that real share prices and corporate investment (as a ratio to the capital stock) are I(1). However, like Debelle and Preston (1995) we have chosen to characterise these series as stationary. Graphical analysis reveals that clear one-off shifts in the levels of these series have occurred. This is confirmed by more sophisticated tests which show that once allowance is made for these shifts, real share prices and investment are better characterised as stationary variables around a broken trend. [15]
Alternatively, the results may simply reflect that speculative share-price movements are distorting the relationship. We address this issue in Section 4.3. [16]
Corporate sales was dropped since it was found to be highly collinear with the output gap term. [17]
With the real return on capital and output gap measures entering Equation 10 contemporaneously, the Hausman (1978) test was employed to test for any simultaneity problems. No simultaneous equation bias was found. Moreover, the results are qualitatively similar if the equation is estimated with instrumental variables, although the output gap term becomes less significant. [18]
To test for simultaneous equation bias, the equation was re-estimated with instrumental variables for the nominal bond yield and inflation. Reassuringly, the results were similar. [19]
This is a particularly strong result given the inherent bias in the estimation process which is that the speculative share-price series will incorporate any misspecification of the fundamental share–price series. That is, the results are biased against finding a relationship between fundamental real share prices and investment. [20]