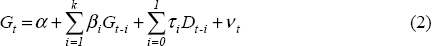RDP 2002-08: Currency Crises and Macroeconomic Performance 2. Some Characteristics of Currency Crises
November 2002
- Download the Paper 136KB
In this section we present some statistics describing the behaviour of output and inflation in the aftermath of a currency crisis. Similar exercises have been undertaken by, inter alia, Bordo and Eichengreen (1999), Hutchison and McDill (1998), and the IMF (1998). The approach in this paper differs from past studies mainly in that it has a greater scope, and that it calculates conditional probabilities of the output and inflation response to a crisis.
We consider a sample of 35 developed and developing countries between 1965 and 1998.[1] Following Kraay (2000), we define a currency crisis as a (nominal) depreciation of the domestic currency against the US dollar of at least 10 per cent in one month. We then eliminate any of these depreciations which were not preceded by a period of exchange rate stability, defined as an average absolute monthly change of less than 2.5 per cent over the past year. This ensures that the currency crises identified are sudden, sharp depreciations. Finally, in order to avoid the double-counting of single prolonged crises, we eliminate any currency crisis which follows a currency crisis within one year. These criteria result in a sample of 78 currency crises.
For each country we construct a time series of the output gap, calculated as the log of real GDP less a Hodrick-Prescott filter estimate of trend.[2] The task then is to identify whether each currency crisis had an effect on output. There are a number of ways that this might be done. Our approach, which has the advantage of being simple, systematic and transparent, is to add a dummy variable representing the currency crisis to an autoregressive model of the output gap. If the coefficient on the dummy variable is statistically significant (at the 10 per cent level), then we conclude that the currency crisis had an effect on output. In order to allow for the possibility of some lagged effect of the currency crisis on output, the precise forms of the regressions estimated are:
for countries with quarterly GDP data and:
for countries with annual GDP data. G represents the output gap and D the dummy variable for the currency crisis. The length of the autoregressive process in each regression is chosen according to the Akaike criterion. The lag lengths on the dummy variable of 4 for the quarterly data regressions and 1 for the annual data regressions are chosen to allow up to a year for the currency crisis to have an effect.
The result of this approach for our sample of countries and currency crises is shown in Table 1. Of the 78 currency crises, 23 (or 29 per cent), are estimated to have had a significant negative effect on output, 5 per cent a positive effect, and 65 per cent no discernible effect.[3] Confidence intervals at 95 per cent for these statistics are also reported. These data suggest that despite boosting external competitiveness, sudden depreciations are more likely to have a negative, rather than positive, effect on output growth.[4]
| Number | Probability | |
|---|---|---|
| Output gap | ||
| Negative | 23 | 0.29 |
| Positive | 4 | 0.05 |
| No effect | 51 | 0.65 |
| Total | 78 | |
| 95 per cent confidence interval | ||
| Negative | 0.19–0.40 | |
| Positive | 0.00–0.10 | |
| No effect | 0.55–0.76 | |
These conclusions can be refined by considering various partitions of the data. For example, Table 2 shows the sub-sample of currency crises which coincided with banking crises.[5] Of these ‘twin crises’, 47 per cent were associated with a fall in output growth (although the sample size is small and the 95 per cent confidence interval extends from 25 per cent to 70 per cent), and there are no positive outcomes. Table 3 divides the sample into higher-income and lower-income countries, based on median per capita income in 1982 (which is the midpoint of our sample's time period). Only 25 per cent of currency crises in higher-income countries had a negative effect on output, compared with 33 per cent for lower-income countries.[6] Since there are as many high-income as low-income countries, but a greater number of crises in low-income countries, poor countries also appear more likely to suffer a crisis.
| Number | Probability | |
|---|---|---|
| Output gap | ||
| Negative | 9 | 0.47 |
| Positive | 0 | 0.00 |
| No effect | 10 | 0.53 |
| Total | 19 | |
| 95 per cent confidence interval | ||
| Negative | 0.25–0.70 | |
| Positive | na | |
| No effect | 0.30–0.75 | |
| Number | Probability | |
|---|---|---|
| Higher-income countries | ||
| Output gap | ||
| Negative | 8.5 | 0.25 |
| Positive | 2.0 | 0.06 |
| No effect | 24.0 | 0.70 |
| Total | 34.5 | |
| 95 per cent confidence interval | ||
| Negative | 0.10–0.39 | |
| Positive | 0.00–0.14 | |
| No effect | 0.54–0.85 | |
| Lower-income countries | ||
| Output gap | ||
| Negative | 14.5 | 0.33 |
| Positive | 2.0 | 0.05 |
| No effect | 27.0 | 0.62 |
| Total | 43.5 | |
| 95 per cent confidence interval | ||
| Negative | 0.19–0.47 | |
| Positive | 0.00–0.11 | |
| No effect | 0.48–0.76 | |
The sample is also divided into relatively open and closed countries (based on the ratio of exports plus imports to GDP in 1982) in Table 4. Unsurprisingly, currency crises are more likely to have no effect in relatively closed economies (73 per cent) than in open economies (59 per cent). Finally, in Table 5, we split the sample into countries with high and low exchange-rate volatility using the standard deviations of the monthly changes in the value of their currencies. Those countries whose currencies have lower than average volatility are less likely to be adversely affected by a currency crisis (20 per cent) than countries whose exchange rates are traditionally more volatile (37 per cent).
| Number | Probability | |
|---|---|---|
| Higher-trade countries | ||
| Output gap | ||
| Negative | 13.5 | 0.33 |
| Positive | 3.5 | 0.09 |
| No effect | 24.0 | 0.59 |
| Total | 41.0 | |
| 95 per cent confidence interval | ||
| Negative | 0.19–0.47 | |
| Positive | 0.00–0.17 | |
| No effect | 0.43–0.74 | |
| Lower-trade countries | ||
| Output gap | ||
| Negative | 9.5 | 0.26 |
| Positive | 0.5 | 0.01 |
| No effect | 27.0 | 0.73 |
| Total | 37.0 | |
| 95 per cent confidence interval | ||
| Negative | 0.12–0.40 | |
| Positive | 0.00–0.05 | |
| No effect | 0.59–0.87 | |
| Number | Probability | |
|---|---|---|
| Higher-volatility countries | ||
| Output gap | ||
| Negative | 16 | 0.37 |
| Positive | 1 | 0.02 |
| No effect | 26 | 0.60 |
| Total | 43 | |
| 95 per cent confidence interval | ||
| Negative | 0.23–0.52 | |
| Positive | 0.00–0.07 | |
| No effect | 0.46–0.75 | |
| Lower-volatility countries | ||
| Output gap | ||
| Negative | 7 | 0.20 |
| Positive | 3 | 0.09 |
| No effect | 25 | 0.71 |
| Total | 35 | |
| 95 per cent confidence interval | ||
| Negative | 0.07–0.33 | |
| Positive | 0.00–0.18 | |
| No effect | 0.56–0.86 | |
The finding that currency crises tend to have a negative effect on output in the short run is common in the literature (e.g. Bordo and Eichengreen (1999); Hutchison and McDill (1998); IMF (1998)), and may be explained in terms of income and substitution effects. The income effect of a sudden depreciation, such as the increase in interest payable on foreign-currency denominated debt, is immediate, whereas the substitution towards domestic goods in response to the lower exchange rate may happen with a lag. Unsurprisingly, a country hit by a banking crisis as well as a currency crisis is more likely to suffer a contraction in output. It is also uncontroversial that exchange rate movements are more likely to affect aggregate output in open economies than closed economies.
The finding that higher-income countries tend to be less adversely affected by currency crises was also expected, for a number of reasons. To begin with, higher-income countries tend to have more robust financial and banking systems, which are better able to handle shocks. Also, as discussed in Hausmann, Panizza and Stein (2000), higher-income countries are more likely to be able to borrow abroad in their own currency, suggesting a lower reliance on foreign-currency-denominated foreign debt. And finally, lower-income countries tend to have higher exchange-rate volatility: 14 of the 17 poorest countries in the sample have high exchange-rate volatility on our criteria.
Turning now to the behaviour of inflation in the aftermath of a currency crisis, Figure 1 depicts the changes in inflation that take place after currency crises. The horizontal axis measures average year-ended CPI inflation in the two years after a currency crisis to the same average in the two years preceding the crisis. So, for example, a ratio of two means that average inflation doubled from the two years before a crisis to the two years after it. The lack of CPI data for some countries and time periods has reduced the sample size from 78 to 59.
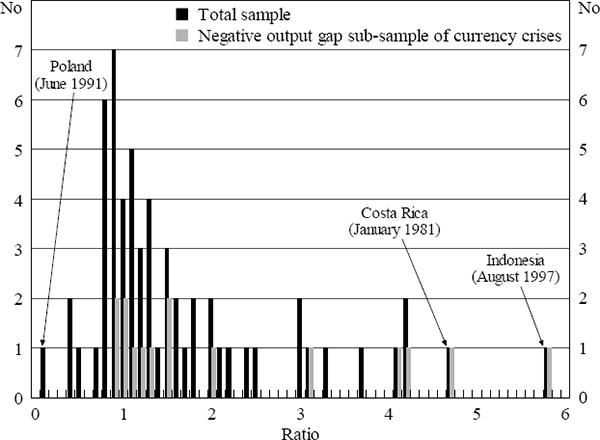
Notes: The figure shows average inflation in the two years following a crisis, divided by average inflation in the two years preceding the crisis. Figures greater than one indicate a rise in inflation.
The distribution is clearly positively skewed. The finding that inflation tends to rise following a currency crisis is not surprising, although it is of some interest that in about 30 per cent of crises, average inflation actually fell. Interestingly, these falls were not confined to countries which experienced output contractions. In fact, average inflation fell in only 2 of the 15 crises in which the output effect was negative. Therefore, at least in our sample, when a currency crisis has an adverse effect, both output and inflation tend to deteriorate.
Table 6 divides the sample according to income, Table 7 according to openness, and Table 8 according to exchange rate variability. Inflation is much more likely to rise substantially in lower-income countries after a currency crisis than in higher-income countries, and in relatively open economies than closed economies. The results of the stable/variable exchange-rate country split are striking. Average inflation in countries accustomed to exchange rate stability rose by at least 50 per cent in only one quarter of all currency crises. The corresponding figure for countries more accustomed to exchange rate variability is about 56 per cent. Once again, this may be reflecting a correlation between income and exchange rate variability.
| Inflation ratio | Number | Proportion |
|---|---|---|
| Higher-income countries | ||
| <0.5 | 2.0 | 0.07 |
| 0.5–0.9 | 8.0 | 0.29 |
| 1.0–1.4 | 9.5 | 0.35 |
| 1.5–2.0 | 5.0 | 0.18 |
| >2.0 | 3.0 | 0.11 |
| Total | 27.5 | |
| Lower-income countries | ||
| <0.5 | 1.0 | 0.03 |
| 0.5–0.9 | 7.0 | 0.22 |
| 1.0–1.4 | 7.5 | 0.24 |
| 1.5–2.0 | 5.0 | 0.16 |
| >2.0 | 11.0 | 0.35 |
| Total | 31.5 | |
| Inflation ratio | Number | Proportion |
|---|---|---|
| Higher-trade countries | ||
| <0.5 | 2.0 | 0.06 |
| 0.5–0.9 | 8.0 | 0.22 |
| 1.0–1.4 | 8.5 | 0.24 |
| 1.5–2.0 | 8.0 | 0.22 |
| >2.0 | 9.5 | 0.26 |
| Total | 36.0 | |
| Lower-trade countries | ||
| <0.5 | 1.0 | 0.04 |
| 0.5–0.9 | 7.0 | 0.30 |
| 1.0–1.4 | 8.5 | 0.37 |
| 1.5–2.0 | 2.0 | 0.09 |
| >2.0 | 4.5 | 0.20 |
| Total | 23.0 | |
| Inflation ratio | Number | Proportion |
|---|---|---|
| Higher-volatility countries | ||
| <0.5 | 1 | 0.04 |
| 0.5–0.9 | 5 | 0.19 |
| 1.0–1.4 | 6 | 0.22 |
| 1.5–2.0 | 7 | 0.26 |
| >2.0 | 8 | 0.30 |
| Total | 27 | |
| Lower-volatility countries | ||
| <0.5 | 2 | 0.06 |
| 0.5–0.9 | 12 | 0.38 |
| 1.0–1.4 | 10 | 0.31 |
| 1.5–2.0 | 3 | 0.09 |
| >2.0 | 5 | 0.16 |
| Total | 32 | |
Two final hypotheses deserve comment. First, it might be thought that the predisposition of lower-income countries to suffer more from currency crises and the correlation between income and exchange rate stability may reflect the tendency of exchange rates in poor countries to depreciate by more in a crisis. Table 9 dispels this idea. It shows the distribution of the 1-month depreciations for our sample of currency crises and reveals no significant difference between higher-income and lower-income countries. Just under two-thirds of currency crises for both groups involved depreciations of 20 per cent or less, although depreciations in lower-income countries were a little more likely to be in the 16–20 per cent band. Depreciations of more than 20 per cent in higher-income and lower-income countries are similarly distributed. Therefore, the different output and inflation responses to currency crises in higher-income and lower-income countries do not appear to be driven by different magnitudes of depreciation.
| Size | Number | Proportion |
|---|---|---|
| Higher-income countries | ||
| 10–15 per cent | 17.5 | 0.51 |
| 16–20 per cent | 4.0 | 0.12 |
| 21–30 per cent | 3.0 | 0.09 |
| 31–40 per cent | 1.0 | 0.03 |
| >40 per cent | 9.0 | 0.26 |
| Total | 34.5 | |
| Lower-income countries | ||
| 10–15 per cent | 18.5 | 0.43 |
| 16–20 per cent | 9.0 | 0.21 |
| 21–30 per cent | 5.0 | 0.11 |
| 31–40 per cent | 2.0 | 0.05 |
| >40 per cent | 9.0 | 0.21 |
| Total | 43.5 | |
Second, there is a common perception that the world has become more ‘crisis prone’ in recent years (Bordo and Eichengreen 1999). Table 10, which shows the timing distribution of our sample of currency crises, provides just a little support for this hypothesis. Apart from a concentration of crises occurring in the early 1980s (which is about the midpoint of our sample), the distribution is fairly evenly spread, with only a slightly higher proportion of crises occurring after the early 1980s than before.
| Timing | Number | Proportion |
|---|---|---|
| 1965–1970 | 9 | 0.12 |
| 1971–1975 | 9 | 0.12 |
| 1976–1980 | 8 | 0.10 |
| 1981–1985 | 23 | 0.29 |
| 1986–1990 | 11 | 0.14 |
| 1991–1995 | 11 | 0.14 |
| 1996–1998 | 7 | 0.09 |
| Total | 78 |
Footnotes
Our sample is that used by Kraay (2000), although a number of crises have been eliminated because of a lack of macroeconomic data needed for our analysis. The countries and timing of each crisis are listed in Appendix A. [1]
Following convention, we set the smoothing parameter in the filter equal to 100 (1,600) for countries with annual (quarterly) GDP data. [2]
We tested the sensitivity of these results to our definition of a currency crisis by changing the ‘10 per cent in one month’ rule to ‘20 per cent in three months’. This did not materially alter the results. [3]
Although currency crises are defined in terms of nominal depreciations, the short (one month) time frame and the presumed stickiness of inflation mean that, in almost all cases, the real exchange rate will also depreciate. [4]
The list and dates of banking crises have been compiled from Caprio and Klingebiel (1996), Demirgiic-Kunt and Detragiache (1997) and Kaminsky and Reinhart (1996). Further details are provided in Appendix A. [5]
Mean per capita income in our sample of countries is substantially higher than median, reflecting the inclusion of a few very rich countries. If mean per capita income is used to divide the sample, 14 per cent of currency crises in higher-income countries and 36 per cent in lower-income countries had a negative effect on output, reinforcing the results in Table 3. [6]