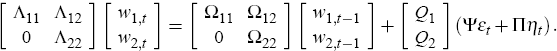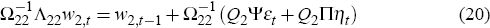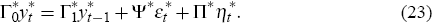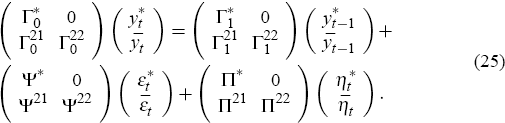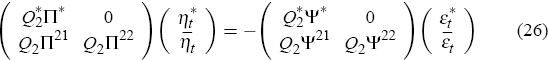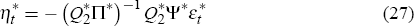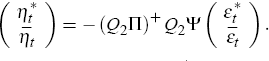RDP 2007-06: The Butterfly Effect of Small Open Economies 3. Stable Rational Expectations Equilibria

3.1 Existence, Uniqueness and Multiplicity

For any variable, say π_t, the expectational error, , is defined as π_t − E_t−1π_t, where E_t−1 is the expectations operator conditional on information at t−1, and satisfies 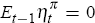for all periods t. As in Sims (2001), we collect the expectational errors in a k × 1 vector η_t and write the model, given by Equations (1) to (18), in matrix form as follows:

where ε_t is a l × 1 vector of fundamental serially uncorrelated random disturbances and the n × 1 vector y_t contains the remaining variables, including conditional expectations.

Lubik and Schorfheide (2003) have extended Sims's (2001) solution for linear rational expectations models by characterising the full set of stable solutions. For completeness we provide a discussion below.

The QZ decomposition (generalised Schur decomposition) yields unitary matrices (complex matrices with orthonormal columns) Q and Z, and upper-triangular matrices Λ and Ω such that Γ₀=Q′ΛZ′ and Γ₁=Q′ΩZ′. An important by-product of this decomposition is that it gives the generalised eigenvalues of Γ₀ and Γ₁ as the ratios of the diagonal elements of the matrices Λ and Ω.^[13]

Next, we define a new set of variables, w_t = Z′y_t, and partition the resulting system into the m variables whose generalised eigenvalues are greater than 1, w_2,t, and those whose generalised eigenvalues are less than 1, w_1,t. Then, in Equation (19), we substitute the matrices Γ₀ and Γ₁ for their QZ decompositions and pre-multiply by Q to obtain

In the expression above, the bottom set of equations, which can be rewritten as

governs the behaviour of the m × 1 vector of purely explosive variables, w_2,t. For the system to be stable, the expectational errors, η_t, have to offset the impact that fundamental shocks, ε_t, have on the purely explosive variables, w_2,t. In other words, a stable solution requires that in all periods t,

Every possible l × 1 vector of fundamental shocks, ε_t, gives rise to a new m × 1 vector −Q₂Ψε_t; and for every possible −Q₂Ψε_t, Equation (21) asserts that there exists a combination of the columns of Q₂Π capable of producing exactly −Q₂Ψε_t. As Sims (2001) shows, a stable solution to Equation (19) exists if, and only if, each of the columns of Q₂Ψ can be obtained as linear combinations of the columns of Q₂Π.^[14]

Note that Q₂Π has dimensions m × k. So there are m equations and k expectational errors to be determined. If the number of explosive variables, m, equals the number of expectational errors, k, and each of the m equations is independent of one another, then Q₂Π is a full rank matrix and the expectational errors are unique linear combinations of the fundamental shocks. That is,

In this case, Equation (19) has a unique solution and the dynamics of y_t are exclusively driven by fundamental shocks.

It is possible, however, that Equation (21) does not determine η_t in a unique manner. For instance, if k exceeds m, Equation (21) can be satisfied for infinitely many values of η_t. In this way, the system admits expectational errors that are unrelated to the fundamental disturbances.

Even in the complete absence of fundamental shocks, expectational errors can become a source of fluctuations. To see this, suppose that k > m and ε_t = 0 for all t. Then Equation (21) reduces to the homogeneous system Q₂Πη_t = 0. Since there are more unknowns than equations, it follows that Q₂Πη_t = 0 has a non-trivial solution (η_t ≠ 0), and, in fact, infinitely many of them. Continue to assume that ε_t = 0 and η_t ≠ 0 for all t, then Equation (19) becomes Γ₀y_t = Γ₁y_t−1 + Πη_t, which explicitly shows that the dynamics of y_t are, in this case, exclusively a function of non-fundamental shocks.

Formally, the variation in η_t that may arise under indeterminacy, and that is unrelated to the variation in ε_t is the result of sunspot shocks, which we denote ξ_t.^[15]

3.2 Expectations and Size

The small open economy model given by Equations (1) to (18) has a particular structure meant to capture the size differences of the two economies. The large economy, described by Equations (1) to (7), can be solved in isolation without reference to any other equation in the system. Thus, the large economy can be written as a self-contained system as follows:

Proceeding as before, the stability condition of the large economy is

Note that if the solution to Equation (24) is unique, the k* foreign expectational errors, 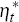, are, exclusively, linear combinations of the l* foreign fundamental shocks, .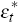

It is possible to partition y_t into the n* foreign variables,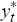, and 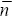remaining ones, ; ε_t and η_t can be partitioned in a similar manner, so that Equation (19) can be written as follows:

The dimensions of the sub-matrices, 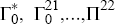, are conformable to the partition. The stability condition in its partitioned form satisfies

and the sub-matrices conform also to the partition obtained from the QZ decomposition: .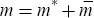

If the equilibrium of the large economy is unique, it follows that

which, in turn, implies that we can solve the second set of equations in Equation (26) as

Equations (27) and (28) highlight an important aspect of the structure of the model: uniqueness in the large economy is sufficient to ensure that its dynamics are driven only by its fundamental shocks, regardless of whether or not the small economy's expectational errors, 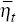, are uniquely determined by its fundamentals. As one would expect, indeterminacy which originates in the small economy does not affect the equilibrium dynamics of the large economy. The converse, as one would also expect, is that indeterminacy caused by the large economy affects the determination of 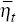and the dynamics of the small economy.

Surprisingly, perhaps, indeterminacy arising in the large economy opens up a channel through which shocks to the small economy, , influence the determination of 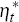, and therefore the dynamics of the large economy. Put differently, foreign indeterminacy allows the small economy's shocks, , to act exactly like non-fundamental shocks for the large economy. The small economy's shocks, , can be described in this way because they do not influence the dynamics of the large economy under uniqueness. This is the ‘butterfly effect’: a situation in which the failure to pin down the equilibrium of the large economy allows developments in the small economy to affect developments in the large one.

To see this mechanism, consider Equation (26), and assume that m* < k* and 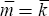. Clearly, indeterminacy in the large economy translates into indeterminacy for the whole system because m < k. In this case, Q₂Π is not an invertible matrix because of the rank deficiency stemming from the large economy's equations. Nevertheless, the full set of solutions can be calculated with the generalised inverse of Q₂Π, which we denote by (Q₂Π)⁺:^[16]

It is important to observe that the decrease in m* caused by foreign indeterminacy (relative to the m* = k* case), removes a set of zero-restrictions from Equation (26) – restrictions that were necessary to isolate the large economy from the small one.

Formally, the vector of expectational errors, η_t, belongs to 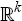. For the sub-vector 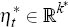to equate to the same value as the one that would have been obtained had we solved for the foreign system (Equation (23)) in isolation, none of the zero-restrictions that show up in the full rank version of Equation (26) can be removed. The ‘butterfly effect’ appears because, in solving for η_t in 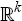, foreign indeterminacy effectively takes away some of the zero-restrictions that were necessary to obtain the same solution for 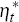that would have been obtained had we solved for the large economy in isolation, in .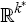

If the large economy is solved in isolation under indeterminacy, the ‘butterfly effect’, of course, can never occur. To justify this approach requires the additional assumption that agents in the large economy form their expectations solely on the basis of information from that economy alone. In this case, the set of multiple solutions of the large economy can be computed with the generalised inverse of . The foreign expectational errors are then given by , in which case , by construction, can never influence 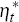.^[17] Solving the large economy first and then using its solution as an exogenous process for the small economy, or solving them simultaneously, is equivalent only if the equilibrium of the large economy is unique.

Thus, uniqueness of the large economy's equilibrium constrains the formation of expectations to a subset of the full information set. ‘Smallness’ is then a property that emerges from the unique determination of the large economy's equilibrium, but not a general property of the system.

Footnotes

A standard eigenvalue problem is of the form Ax = λx. A generalised eigenvalue problem takes the form Ax = μBx. The values of μ that satisfy this last equation are called the generalised eigenvalues of A and B. [13]

Sims's condition is actually more general because it allows any pattern of serial correlation in ε_t. The condition reduces to Equation (21) under our assumption of serially uncorrelated disturbances. Unless otherwise stated, the existence condition given by Equation (21) always holds in our analysis. Stability also requires the initial condition w_2,0 = 0. [14]

See Lubik and Schorfheide (2003) for an expression of the expectational errors as linear combinations of fundamental shocks, ε_t, and non-fundamental shocks, ξ_t. The only restriction on the distribution of sunspots shocks is that they follow a martingale difference sequence; that is, E_t–1ξ_t = 0. [15]

The solution shown above is the minimum distance solution which satisfies Equation (21). The general solution to the inhomogeneous system Q₂Πη_t = −Q₂Ψε_t is the sum of a particular solution of the inhomogeneous system and the general solution of the corresponding homogeneous system Q₂Πη_t = 0. Under the assumption that Q₂Π is a full row rank matrix, (Q₂Π(Q₂Π)′)⁻¹ exists. Then the solution η_t can always be written as the sum of the generalised inverse solution of the inhomogeneous system and a solution of the homogeneous system: η_t = −(Q₂Π)⁺Q₂Ψε_t + (I − (Q₂Π)′(Q₂Π(Q₂Π)′)⁻¹Q₂Π)z_t, where the vector z_t is arbitrary (apart from its dimensionality). [16]

The solution shown here is the minimum distance solution which satisfies Equation (24). The general solution to the inhomogeneous system given by Equation (24) is the sum of a particular solution of the inhomogeneous system and the general solution of the corresponding homogeneous system 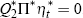. [17]