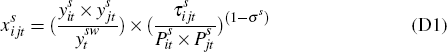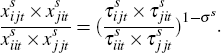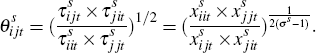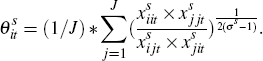RDP 2014-07: International Trade Costs, Global Supply Chains and Value-added Trade in Australia Appendix D: Measures of International Trade Costs

International trade costs are estimated using the following gravity equation:

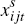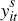where, for each industry s in year t, denotes exports from country i to country j, and 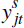denote the levels of output produced in country i and country j respectively, denotes world output, and 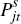are the aggregate price indices (or ‘multilateral resistance’ terms) of country i and country j respectively, 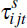is the bilateral trade cost, σ^s > 1 is the elasticity of substitution across goods within the industry.

The aggregate price indices measure the average trade barriers imposed by country i and country j. All else being equal, bilateral trade between country i and country j increases if either country i or country j raise their average trade barriers. This is because, for a given bilateral trade barrier between country i and country j, higher barriers between the importing country j and its other trading partners reduce the relative price of exports from country i to country j. But if the exporting country i also lifts its barriers with all trading partners, this lowers aggregate demand for its exports and therefore reduces its supply price in equilibrium. For a given bilateral trade barrier between country i and country j, this raises the level of trade between the two countries (Anderson and van Wincoop 2003).

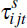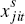We cannot directly solve Equation (D1) for the trade cost term () because the aggregate price indices are not observed. However, they can be eliminated by multiplying the gravity equation by its counterpart for trade flows in the opposite direction () and then dividing it by the product of the gravity equations for domestic trade flows in each country :

The geometric average of trade costs between the two countries is then given by:

To obtain aggregate international trade costs for each industry and year we take a simple (unweighted) mean of trade costs across all trading partners:

The ad valorem equivalent of international trade costs is calculated by subtracting the value of one from this expression.