RDP 9108: Australia's Real Exchange Rate – Is it Explained by the Terms of Trade or by Real Interest Differentials? 2. The Stylised Facts
September 1991
- Download the Paper 531KB
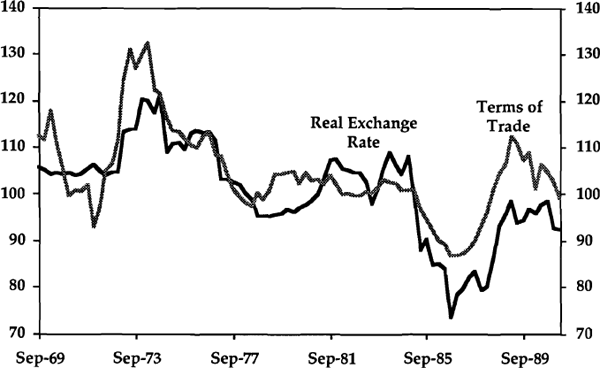
Graph 1 shows Australia's trade-weighted real exchange rate and the terms of trade for goods and services from the December quarter 1969 to the March quarter 1991.[2] There appears to be a clear correlation between movements in the terms of trade and the real exchange rate. Three periods stand out for special mention. Firstly, between 1978 and 1984, the real exchange rate appreciated and was considerably more volatile than the slowly falling terms of trade. Secondly, although in proportionate terms the real exchange rate fell more than the terms of trade between early 1984 and mid-1986, it appreciated less than proportionately in the subsequent four years. Finally, the terms of trade fell 10% from its average level in 1989 to its level in the March quarter 1991. By contrast, the real exchange rate fell 3.5% from its average level in 1989 to its average level in the March quarter 1991, and, by the end of March 1991, was back to its average 1989 level.
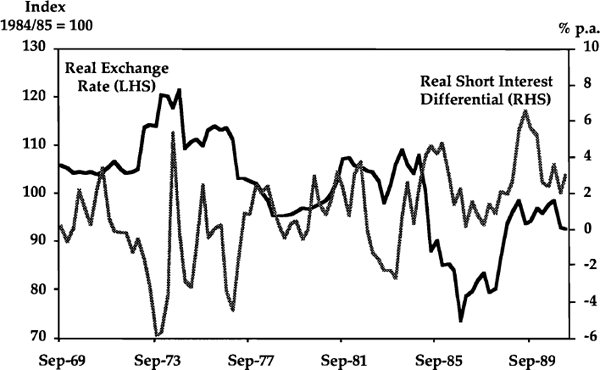
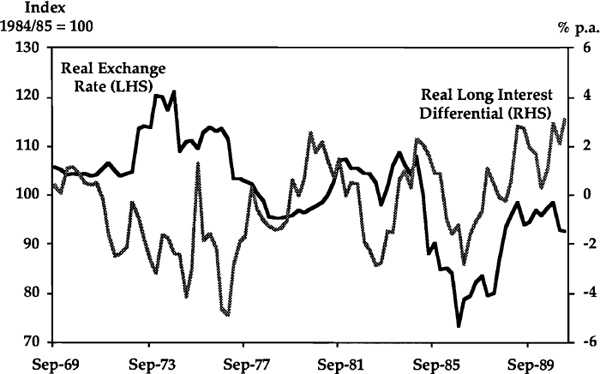
Graphs 2 and 3 show Australia's real exchange rate along with the short and long real interest differentials respectively. The real interest differentials are calculated as the difference between Australian real rates and an arithmetic average of real rates in the US, UK, Japan and Germany, using actual inflation over the past 12 months as a proxy for expected inflation. Over the 1970s, these series do not appear to move with the real exchange rate. However, since the early 1980s the real exchange rate appears to be more closely correlated with real interest differentials (especially with the long real differential). These graphs provide some evidence that in the most recent period, relatively high Australian real interest rates have tended to keep the real exchange rate high.
(a) Correlation Analysis
Table 1 shows the correlations between the variables used to explain the real exchange rate for two different sample periods. As expected, the Table shows that the real exchange rate is positively correlated with the terms of trade both over the whole sample period and since the float. It also shows that while the real exchange rate is negatively correlated with long and short real interest differentials over the whole sample period, it is positively correlated with both since the float. Note that the correlation is stronger with long differentials than with short ones after the float. Post-float, the terms of trade is also correlated with real interest differentials – and again the correlation is stronger with long real differentials than with short ones.
| RER |
Terms of Trade |
Short Interest Differential |
Long Interest Differential |
|
|---|---|---|---|---|
| 1969:4 to 1990:4 | ||||
| RER | 1.00 | 0.78 | −0.42 | −0.27 |
| Terms of Trade | 1.00 | −0.40 | −0.17 | |
| Short Interest Differential | 1.00 | 0.75 | ||
| 1984:1 to 1990:4 | ||||
| RER | 1.00 | 0.77 | 0.26 | 0.63 |
| Terms of Trade | 1.00 | 0.54 | 0.76 | |
| Short Interest Differential | 1.00 | 0.66 | ||
Footnote
All the graphs in the paper show variables in levels. By contrast, in all the econometric estimation, the real exchange rate and measures of the terms of trade are used in log form. We have taken considerable care to construct an accurate measure of Australia's real exchange rate. Our measure is a trade-weighted exchange rate adjusted ratio of the Australian “Medicare-adjusted” CPI to the CPIs of its 22 major trading partners. Trade weights are calculated as an average of annual trade flows from 1980 to 1989. All exchange rates are quarterly averages. See the Data Appendix for further detail. [2]